Math Is Fun Forum
You are not logged in.
- Topics: Active | Unanswered
#1 This is Cool » Phosphoric Acid » Yesterday 22:32:07
- Jai Ganesh
- Replies: 0
Phosphoric Acid
Gist
Phosphoric acid (H3PO4) is a weak mineral acid used in food, agriculture, and industry, and it is found as a clear liquid or white crystalline solid. In foods, it's used as an acidulant and preservative, while in fertilizers, it promotes plant growth. Due to its corrosive nature, concentrated solutions require protective gear to avoid skin, eye, and respiratory irritation.
Phosphoric acid has numerous uses, most notably in the production of phosphate fertilizers. It is also used in the food and beverage industry as an acidic flavoring agent and preservative, particularly in soft drinks. Other common applications include metal treatment, cleaning products, water treatment, detergents, and in the manufacturing of some pharmaceuticals and personal care products.
Summary
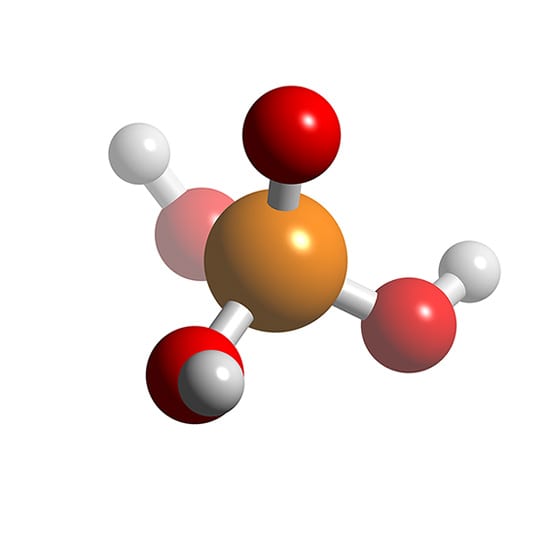
Phosphoric acid (orthophosphoric acid, monophosphoric acid or phosphoric(V) acid) is a colorless, odorless phosphorus-containing solid, and inorganic compound with the chemical formula H3PO4. It is commonly encountered as an 85% aqueous solution, which is a colourless, odourless, and non-volatile syrupy liquid. It is a major industrial chemical, being a component of many fertilizers.
The name "orthophosphoric acid" can be used to distinguish this specific acid from other "phosphoric acids", such as pyrophosphoric acid. Nevertheless, the term "phosphoric acid" often means this specific compound; and that is the current IUPAC nomenclature.
Purification
Phosphoric acid produced from phosphate rock or thermal processes often requires purification. A common purification method is liquid–liquid extraction, which involves the separation of phosphoric acid from water and other impurities using organic solvents, such as tributyl phosphate (TBP), methyl isobutyl ketone (MIBK), or n-octanol. Nanofiltration involves the use of a premodified nanofiltration membrane, which is functionalized by a deposit of a high molecular weight polycationic polymer of polyethyleneimines. Nanofiltration has been shown to significantly reduce the concentrations of various impurities, including cadmium, aluminum, iron, and rare earth elements. The laboratory and industrial pilot scale results showed that this process allows the production of food-grade phosphoric acid.
Fractional crystallization can achieve higher purities typically used for semiconductor applications. Usually a static crystallizer is used. A static crystallizer uses vertical plates, which are suspended in the molten feed and which are alternatingly cooled and heated by a heat transfer medium. The process begins with the slow cooling of the heat transfer medium below the freezing point of the stagnant melt. This cooling causes a layer of crystals to grow on the plates. Impurities are rejected from the growing crystals and are concentrated in the remaining melt. After the desired fraction has been crystallized, the remaining melt is drained from the crystallizer. The purer crystalline layer remains adhered to the plates. In a subsequent step, the plates are heated again to liquify the crystals and the purified phosphoric acid drained into the product vessel. The crystallizer is filled with feed again and the next cooling cycle is started.
Details
Phosphoric acid, (H3PO4) is the most important oxygen acid of phosphorus, used to make phosphate salts for fertilizers. It is also used in dental cements, in the preparation of albumin derivatives, and in the sugar and textile industries. It serves as an acidic, fruitlike flavouring in food products.
Pure phosphoric acid is a crystalline solid (melting point 42.35° C, or 108.2° F); in less concentrated form it is a colourless syrupy liquid. The crude acid is prepared from phosphate rock, while acid of higher purity is made from white phosphorus.
Phosphoric acid forms three classes of salts corresponding to replacement of one, two, or three hydrogen atoms. Among the important phosphate salts are: sodium dihydrogen phosphate (NaH2PO4), used for control of hydrogen ion concentration (acidity) of solutions; disodium hydrogen phosphate (Na2HPO4), used in water treatment as a precipitant for highly charged metal cations; trisodium phosphate (Na3PO4), used in soaps and detergents; calcium dihydrogen phosphate or calcium superphosphate (Ca[H2PO4]2), a major fertilizer ingredient; calcium monohydrogen phosphate (CaHPO4), used as a conditioning agent for salts and sugars.
Phosphoric acid molecules interact under suitable conditions, often at high temperatures, to form larger molecules (usually with loss of water). Thus, diphosphoric, or pyrophosphoric, acid (H4P2O7) is formed from two molecules of phosphoric acid, less one molecule of water. It is the simplest of a homologous series of long chain molecules called polyphosphoric acids, with the general formula H(HPO3)nOH, in which n = 2, 3, 4, . . . . Metaphosphoric acids, (HPO3)n, in which n = 3, 4, 5, . . ., are another class of polymeric phosphoric acids. The known metaphosphoric acids are characterized by cyclic molecular structures. The term metaphosphoric acid is used also to refer to a viscous, sticky substance that is a mixture of both long chain and ring forms of (HPO3)n. The various polymeric forms of phosphoric acid are also prepared by hydration of phosphorus oxides.
Additional Information
Phosphoric acid, also known as orthophosphoric acid, is a chemical compound. It is also an acid. Its chemical formula is H3PO4. It contains hydrogen and phosphate ions. Its official IUPAC name is trihydroxidooxidophosphorus.
Properties
Phosphoric acid is a white solid. It melts easily to make a viscous liquid. It tastes sour when diluted (mixed with a lot of water). It can be deprotonated three times. It is very strong, although not as much as the other acids like hydrochloric acid. It does not have any odor. It is corrosive when concentrated. Salts of phosphoric acid are called phosphates.
Preparation
Phosphoric acid can be made by dissolving phosphorus(V) oxide in water. This makes a very pure phosphoric acid that is good for food. A less pure form is made by reacting sulfuric acid with phosphate rock. This can be purified to make food-grade phosphoric acid if needed.
Uses
It is used to make sodas sour. It is also used when a nonreactive acid is needed. It can be used to make hydrogen halides, such as hydrogen chloride. Phosphoric acid is heated with a sodium halide to make the hydrogen halide and sodium phosphate. It is used to react with rust to make black iron(III) phosphate, which can be scraped off, leaving pure iron. It can be used to clean teeth.
There are many minor uses of phosphoric acid. Phosphoric acid with a certain isotope of phosphorus is used for nuclear magnetic resonance. It is also used as an electrolyte in some fuel cells. It can be used as a flux. It can etch certain things in semiconductor making.
Safety
Phosphoric acid is one of the least toxic acids. When it is diluted, it just has a sour taste. When it is concentrated, it can corrode metals.
#2 Re: Dark Discussions at Cafe Infinity » crème de la crème » Yesterday 17:29:43
2366) Luis Federico Leloir
Gist:
Work
Carbohydrates, including sugars and starches, are of paramount importance to the life processes of organisms. Luis Leloir demonstrated that nucleotides—molecules that also constitute the building blocks of DNA molecules—are crucial when carbohydrates are generated and converted. In 1949 Leloir discovered that one type of sugar’s conversion to another depends on a molecule that consists of a nucleotide and a type of sugar. He later showed that the generation of carbohydrates is not an inversion of metabolism, as had been assumed previously, but processes with other steps.
Summary
Luis Federico Leloir (born Sept. 6, 1906, Paris, France—died Dec. 2, 1987, Buenos Aires, Arg.) was an Argentine biochemist who won the Nobel Prize for Chemistry in 1970 for his investigations of the processes by which carbohydrates are converted into energy in the body.
After serving as an assistant at the Institute of Physiology, University of Buenos Aires, from 1934 to 1935, Leloir worked a year at the biochemical laboratory at the University of Cambridge and in 1937 returned to the Institute of Physiology, where he undertook investigations of the oxidation of fatty acids. In 1947 he obtained financial support to set up the Institute for Biochemical Research, Buenos Aires, where he began research on the formation and breakdown of lactose, or milk sugar, in the body. That work ultimately led to his discovery of sugar nucleotides, which are key elements in the processes by which sugars stored in the body are converted into energy. He also investigated the formation and utilization of glycogen and discovered certain liver enzymes that are involved in its synthesis from glucose.
Details
Luis Federico Leloir (September 6, 1906 – December 2, 1987) was an Argentine physician and biochemist who received the 1970 Nobel Prize in Chemistry for his discovery of the metabolic pathways by which carbohydrates are synthesized and converted into energy in the body. Although born in France, Leloir received the majority of his education at the University of Buenos Aires and was director of the private research group Fundación Instituto Campomar until his death in 1987. His research into sugar nucleotides, carbohydrate metabolism, and renal hypertension garnered international attention and led to significant progress in understanding, diagnosing and treating the congenital disease galactosemia. Leloir is buried in La Recoleta Cemetery, Buenos Aires.
Biography:
Early years
Leloir's parents, Federico Augusto Rufino and Hortencia Aguirre de Leloir, traveled from Buenos Aires to Paris in the middle of 1906 with the intention of treating Federico's illness. However, Federico died in late August, and a week later Luis was born in an old house at 81 Víctor Hugo Road in Paris, a few blocks away from the Arc de Triomphe. After returning to Argentina in 1908, Leloir lived together with his eight siblings on their family's extensive property El Tuyú that his grandparents had purchased after their immigration from the Basque Country of northern Spain: El Tuyú comprises 400 {km}^{2} of sandy land along the coastline from San Clemente del Tuyú to Mar de Ajó which has since become a popular tourist attraction.
During his childhood, the future Nobel Prize winner found himself observing natural phenomena with particular interest; his schoolwork and readings highlighted the connections between the natural sciences and biology. His education was divided between Escuela General San Martín (primary school), Colegio Lacordaire (secondary school), and for a few months at Beaumont College in England. His grades were unspectacular, and his first stint in college ended quickly when he abandoned his architectural studies that he had begun in Paris' École Polytechnique.
It was during the 1920s that Leloir invented salsa golf (golf sauce). After being served prawns with the usual sauce during lunch with a group of friends at the Ocean Club in Mar del Plata, Leloir came up with a peculiar combination of ketchup and mayonnaise to spice up his meal. With the financial difficulties that later plagued Leloir's laboratories and research, he would joke, "If I had patented that sauce, we'd have a lot more money for research right now.
Nobel Prize
On December 2, 1970, Leloir received the Nobel Prize for Chemistry from the King of Sweden for his discovery of the metabolic pathways in lactose, becoming only the third Argentine to receive the prestigious honor in any field at the time. In his acceptance speech at Stockholm, he borrowed from Winston Churchill's famous 1940 speech to the House of Commons and remarked, "never have I received so much for so little". Leloir and his team reportedly celebrated by drinking champagne from test tubes, a rare departure from the humility and frugality that characterized the atmosphere of Fundación Instituto Campomar under Leloir's direction. The $80,000 prize money was spent directly on research, and when asked about the significance of his achievement, Leloir responded:
"This is only one step in a much larger project. I discovered (no, not me: my team) the function of sugar nucleotides in cell metabolism. I want others to understand this, but it is not easy to explain: this is not a very noteworthy deed, and we hardly know even a little."

#3 Re: This is Cool » Miscellany » Yesterday 16:56:15
2419) Carbon Tetrachloride
Gist
Carbon tetrachloride (CCl4) is a synthetic, non-flammable, colorless liquid with a sweet odor. It was historically used in cleaning products, fire extinguishers, and as a precursor for refrigerants, but its use has been significantly reduced due to its high toxicity. It is harmful to the liver, kidneys, and central nervous system, is considered a suspected human carcinogen, and also depletes the ozone layer.
Carbon tetrachloride (CCl4) has historically been used as a solvent, a cleaning agent, and in fire extinguishers, but its use has been largely phased out due to severe health and environmental concerns. Its primary modern use is as a feedstock for producing other chemicals, such as refrigerants, and it has a few niche industrial and laboratory applications.
Summary
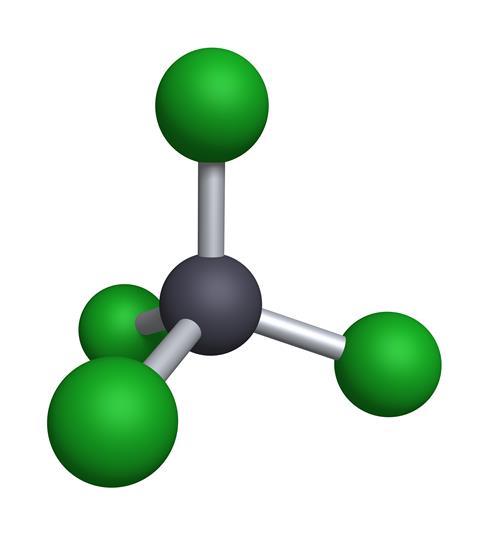
Carbon tetrachloride, also known by many other names (such as carbon tet for short and tetrachloromethane, also recognised by the IUPAC), is a chemical compound with the chemical formula CCl4. It is a non-flammable, dense, colourless liquid with a chloroform-like sweet odour that can be detected at low levels. It was formerly widely used in fire extinguishers, as a precursor to refrigerants, an anthelmintic and a cleaning agent, but has since been phased out because of environmental and safety concerns. Exposure to high concentrations of carbon tetrachloride can affect the central nervous system and degenerate the liver and kidneys. Prolonged exposure can be fatal.
Properties
In the carbon tetrachloride molecule, four chlorine atoms are positioned symmetrically as corners in a tetrahedral configuration joined to a central carbon atom by single covalent bonds. Because of this symmetric geometry, CCl4 is non-polar. Methane gas has the same structure, making carbon tetrachloride a halomethane. As a solvent, it is well suited to dissolving other non-polar compounds such as fats and oils. It can also dissolve iodine. It is volatile, giving off vapors with an odor characteristic of other chlorinated solvents, somewhat similar to the tetrachloroethylene odor reminiscent of dry cleaners' shops.
With a specific gravity greater than 1, carbon tetrachloride will be present as a dense nonaqueous phase liquid if sufficient quantities are spilt in the environment.
Details
Carbon tetrachloride is a manufactured chemical that does not occur naturally. It is a clear liquid with a sweet smell that can be detected at low levels. It is also called carbon chloride, methane tetrachloride, perchloromethane, tetrachloroethane, or benziform. Carbon tetrachloride is most often found in the air as a colorless gas. It is not flammable and does not dissolve in water very easily. It was used in the production of refrigeration fluid and propellants for aerosol cans, as a pesticide, as a cleaning fluid and degreasing agent, in fire extinguishers, and in spot removers. Because of its harmful effects, these uses are now banned and it is only used in some industrial applications.
Carbon tetrachloride appears as a clear colorless liquid with a characteristic odor. Denser than water (13.2 lb / gal) and insoluble in water. Noncombustible. May cause illness by inhalation, skin absorption and/or ingestion. Used as a solvent, in the manufacture of other chemicals, as an agricultural fumigant, and for many other uses.
Carbon tetrachloride may be found in both ambient outdoor and indoor air. The primary effects of carbon tetrachloride in humans are on the liver, kidneys, and central nervous system (CNS). Human symptoms of acute (short-term) inhalation and oral exposures to carbon tetrachloride include headache, weakness, lethargy, nausea, and vomiting. Acute exposures to higher levels and chronic (long-term) inhalation or oral exposure to carbon tetrachloride produces liver and kidney damage in humans. Human data on the carcinogenic effects of carbon tetrachloride are limited. Studies in animals have shown that ingestion of carbon tetrachloride increases the risk of liver cancer. EPA has classified carbon tetrachloride as a Group B2, probable human carcinogen.
Additional Information
Carbon tetrachloride is a colourless, dense, highly toxic, volatile, nonflammable liquid possessing a characteristic odour and belonging to the family of organic halogen compounds, used principally in the manufacture of dichlorodifluoromethane (a refrigerant and propellant).
First prepared in 1839 by the reaction of chloroform with chlorine, carbon tetrachloride is manufactured by the reaction of chlorine with carbon disulfide or with methane. The process with methane became dominant in the United States in the 1950s, but the process with carbon disulfide remains important in countries where natural gas (the principal source of methane) is not plentiful. Carbon tetrachloride boils at 77° C (171° F) and freezes at -23° C (-9° F); it is much denser than water, in which it is practically insoluble.
Formerly used as a dry-cleaning solvent, carbon tetrachloride has been almost entirely displaced from this application by tetrachloroethylene, which is much more stable and less toxic.
#4 Dark Discussions at Cafe Infinity » Club Quotes - IV » Yesterday 16:31:39
- Jai Ganesh
- Replies: 0
Club Quotes - IV
1. My main idea was to create a sports facility for the basics. This is why I established the club. - Sergei Bubka
2. Today I have 35 people who work in the club and associated businesses. - Sergei Bubka
3. We started with that, basically to help kids, and then we created a pole vault school, which is part of the club and exists to this day. The club and school exist. - Sergei Bubka
4. When I was 4 my mother got divorced and we were very close to each other. I always wanted to be with her. She took me everywhere. When she went for dinner with friends or when they had meetings at the tennis club, I was always there. - Martina Hingis
5. I don't belong to any clubs, and I dislike club mentality of any kind, even feminism - although I do relate to the purpose and point of feminism. More in the work of older feminists, really, like Germaine Greer. - Jane Campion
6. I grew up a little girl in the Soviet Union playing at a small sports club. Tennis gave me my life. - Anna Kournikova
7. I have been running maths clubs for children completely free. In my building in Bangalore, I conduct maths clubs for several months, and every child who attended the club was poor in mathematics and is now showing brilliant results. - Shakuntala Devi
8. I've played under some of the biggest and best managers and achieved almost everything in football. Of course it hurts when people question it, but I've come to the end of my career and can look back and say I've achieved everything with every club that I've played for. - David Beckham.
#5 Science HQ » Lawrencium » Yesterday 16:01:50
- Jai Ganesh
- Replies: 0
Lawrencium
Gist
Lawrencium (Lr) is a synthetic, radioactive element with atomic number 103, belonging to the actinide series. It is a highly reactive metal that does not occur naturally and is only produced in tiny quantities for scientific research. Due to its instability, it has a short half-life, though the longest-lived isotope, \(Lr\)-262, has a half-life of about 3.6 hours. It was named after Ernest Lawrence, the inventor of the cyclotron.
Lawrencium has no large-scale commercial or industrial uses because it is a synthetic, highly radioactive element produced in only tiny quantities. Its primary and sole use is for scientific research, where it helps scientists study superheavy elements, nuclear reactions, and electron configurations in laboratory settings.
Summary
Lawrencium is a synthetic chemical element; it has symbol Lr (formerly Lw) and atomic number 103. It is named after Ernest Lawrence, inventor of the cyclotron, a device that was used to discover many artificial radioactive elements. A radioactive metal, lawrencium is the eleventh transuranium element, the third transfermium, and the last member of the actinide series. Like all elements with atomic number over 100, lawrencium can only be produced in particle accelerators by bombarding lighter elements with charged particles. Fourteen isotopes of lawrencium are currently known; the most stable is 266Lr with half-life 11 hours, but the shorter-lived 260Lr (half-life 2.7 minutes) is most commonly used in chemistry because it can be produced on a larger scale.
Chemistry experiments confirm that lawrencium behaves as a heavier homolog to lutetium in the periodic table, and is a trivalent element. It thus could also be classified as the first of the 7th-period transition metals. Its electron configuration is anomalous for its position in the periodic table, having an s2p configuration instead of the s2d configuration of its homolog lutetium. However, this does not appear to affect lawrencium's chemistry.
In the 1950s, 1960s, and 1970s, many claims of the synthesis of element 103 of varying quality were made from laboratories in the Soviet Union and the United States. The priority of the discovery and therefore the name of the element was disputed between Soviet and American scientists. The International Union of Pure and Applied Chemistry (IUPAC) initially established lawrencium as the official name for the element and gave the American team credit for the discovery; this was reevaluated in 1992, giving both teams shared credit for the discovery but not changing the element's name.
Details
Lawrencium (Lr) is a synthetic chemical element, the 14th member of the actinoid series of the periodic table, atomic number 103. Not occurring in nature, lawrencium (probably as the isotope lawrencium-257) was first produced (1961) by chemists Albert Ghiorso, T. Sikkeland, A.E. Larsh, and R.M. Latimer at the University of California, Berkeley, by bombarding a mixture of the longest-lived isotopes of californium (atomic number 98) with boron ions (atomic number 5) accelerated in a heavy-ion linear accelerator. The element was named after American physicist Ernest O. Lawrence. A team of Soviet scientists at the Joint Institute for Nuclear Research in Dubna discovered (1965) lawrencium-256 (26-second half-life), which the Berkeley group later used in a study with approximately 1,500 atoms to show that lawrencium behaves more like the tripositive elements in the actinoid series than like predominantly dipositive nobelium (atomic number 102). The longest-lasting isotope, lawrencium-262, has a half-life of about 3.6 hours.
Element Properties
atomic number : 103
stablest isotope : 262
oxidation state : +3.
Additional Information
The element is named after Ernest Lawrence, who invented the cyclotron particle accelerator. This was designed to accelerate sub-atomic particles around a circle until they have enough energy to smash into an atom and create a new atom. This image is based on the abstract particle trails produced in a cyclotron.
Appearance
A radioactive metal of which only a few atoms have ever been created.
Uses
Lawrencium has no uses outside research.
Biological role
Lawrencium has no known biological role.
Natural abundance
Lawrencium does not occur naturally. It is produced by bombarding californium with boron.
#6 Re: Jai Ganesh's Puzzles » General Quiz » Yesterday 15:22:28
Hi,
#10613. What does the term in Biology Desmosome mean?
#10614. What does the term in Biology Developmental biology mean?
#7 Re: Jai Ganesh's Puzzles » English language puzzles » Yesterday 14:54:02
Hi,
#5809. What does the noun hoard mean?
#5810. What does the noun hoax mean?
#8 Re: Jai Ganesh's Puzzles » Doc, Doc! » Yesterday 14:41:07
Hi,
#2497. What does the medical term Ophthalmoscopy mean?
#9 Re: Jai Ganesh's Puzzles » 10 second questions » Yesterday 14:29:07
Hi,
#9766.
#10 Re: Jai Ganesh's Puzzles » Oral puzzles » Yesterday 14:06:59
Hi,
#6272.
#11 Jokes » Apple Jokes - I » Yesterday 13:51:08
- Jai Ganesh
- Replies: 0
Q: What do you get if you cross an apple with a shellfish?
A: A crab apple !
* * *
Q: Why did Eve want to leave the garden of Eden and move to New York ?
A: She fell for the Big Apple !
* * *
Q: What type of a computer does a horse like to eat?
A Macintosh.
* * *
Q: Why did the farmer hang raincoats all over his orchard?
A: Someone told him he should get an apple Mac.
* * *
Q: How do you make an apple turnover?
A: Push it down hill.
* * *
#12 Re: Exercises » Compute the solution: » Yesterday 13:43:38
Hi,
2616.
#13 This is Cool » Ammonia » 2025-10-15 19:14:17
- Jai Ganesh
- Replies: 0
Ammonia
Gist
Ammonia (NH3 is a colorless, pungent gas composed of nitrogen and hydrogen, commonly used in household cleaners and fertilizers. It is a natural byproduct in the human body from protein breakdown but can be toxic at high concentrations. Ammonia's properties include being highly soluble in water, having a strong odor, and being highly alkaline.
Ammonia is used primarily in agriculture to make fertilizers, and also for industrial purposes like producing plastics, dyes, explosives, and synthetic fibers. It is also found in household products like glass and surface cleaners, and used in applications such as refrigeration and as a fuel for some rockets.
Summary
Ammonia is an inorganic chemical compound of nitrogen and hydrogen with the formula NH3. A stable binary hydride and the simplest pnictogen hydride, ammonia is a colourless gas with a distinctive pungent smell. It is widely used in fertilizers, refrigerants, explosives, cleaning agents, and is a precursor for numerous chemicals. Biologically, it is a common nitrogenous waste, and it contributes significantly to the nutritional needs of terrestrial organisms by serving as a precursor to fertilisers. Around 70% of ammonia produced industrially is used to make fertilisers in various forms and composition, such as urea and diammonium phosphate. Ammonia in pure form is also applied directly into the soil.
Ammonia, either directly or indirectly, is also a building block for the synthesis of many chemicals. In many countries, it is classified as an extremely hazardous substance. Ammonia is toxic, causing damage to cells and tissues. For this reason it is excreted by most animals in the urine, in the form of dissolved urea.
Ammonia is produced biologically in a process called nitrogen fixation, but even more is generated industrially by the Haber process. The process helped revolutionize agriculture by providing cheap fertilizers. The global industrial production of ammonia in 2021 was 235 million tonnes. Industrial ammonia is transported by road in tankers, by rail in tank wagons, by sea in gas carriers, or in cylinders. Ammonia occurs in nature and has been detected in the interstellar medium.
Ammonia boils at −33.34 °C (−28.012 °F) at a pressure of one atmosphere, but the liquid can often be handled in the laboratory without external cooling. Household ammonia or ammonium hydroxide is a solution of ammonia in water.
Details
Ammonia (NH3) is a colourless, pungent gas composed of nitrogen and hydrogen. It is the simplest stable compound of these elements and serves as a starting material for the production of many commercially important nitrogen compounds.
Uses of ammonia
The major use of ammonia is as a fertilizer. In the United States, it is usually applied directly to the soil from tanks containing the liquefied gas. The ammonia can also be in the form of ammonium salts, such as ammonium nitrate, NH4NO3, ammonium sulfate, (NH4)2SO4, and various ammonium phosphates. Urea, (H2N)2C=O, is the most commonly used source of nitrogen for fertilizer worldwide. Ammonia is also used in the manufacture of commercial explosives (e.g., trinitrotoluene [TNT], nitroglycerin, and nitrocellulose).
In the textile industry, ammonia is used in the manufacture of synthetic fibres, such as nylon and rayon. In addition, it is employed in the dyeing and scouring of cotton, wool, and silk. Ammonia serves as a catalyst in the production of some synthetic resins. More important, it neutralizes acidic by-products of petroleum refining, and in the rubber industry it prevents the coagulation of raw latex during transportation from plantation to factory. Ammonia also finds application in both the ammonia-soda process (also called the Solvay process), a widely used method for producing soda ash, and the Ostwald process, a method for converting ammonia into nitric acid.
Ammonia is used in various metallurgical processes, including the nitriding of alloy sheets to harden their surfaces. Because ammonia can be decomposed easily to yield hydrogen, it is a convenient portable source of atomic hydrogen for welding. In addition, ammonia can absorb substantial amounts of heat from its surroundings (i.e., one gram of ammonia absorbs 327 calories of heat), which makes it useful as a coolant in refrigeration and air-conditioning equipment. Finally, among its minor uses is inclusion in certain household cleansing agents.
Preparation of ammonia
Pure ammonia was first prepared by English physical scientist Joseph Priestley in 1774, and its exact composition was determined by French chemist Claude-Louis Berthollet in 1785. Ammonia is consistently among the top five chemicals produced in the United States. The chief commercial method of producing ammonia is by the Haber-Bosch process, which involves the direct reaction of elemental hydrogen and elemental nitrogen.
N2 + 3H2 → 2NH3
This reaction requires the use of a catalyst, high pressure (100–1,000 atmospheres), and elevated temperature (400–550 °C [750–1020 °F]). Actually, the equilibrium between the elements and ammonia favours the formation of ammonia at low temperature, but high temperature is required to achieve a satisfactory rate of ammonia formation. Several different catalysts can be used. Normally the catalyst is iron containing iron oxide. However, both magnesium oxide on aluminum oxide that has been activated by alkali metal oxides and ruthenium on carbon have been employed as catalysts. In the laboratory, ammonia is best synthesized by the hydrolysis of a metal nitride.
Mg3N2 + 6H2O → 2NH3 + 3Mg(OH)2
Physical properties of ammonia
Ammonia is a colourless gas with a sharp, penetrating odour. Its boiling point is −33.35 °C (−28.03 °F), and its freezing point is −77.7 °C (−107.8 °F). It has a high heat of vaporization (23.3 kilojoules per mole at its boiling point) and can be handled as a liquid in thermally insulated containers in the laboratory. (The heat of vaporization of a substance is the number of kilojoules needed to vaporize one mole of the substance with no change in temperature.) The ammonia molecule has a trigonal pyramidal shape with the three hydrogen atoms and an unshared pair of electrons attached to the nitrogen atom. It is a polar molecule and is highly associated because of strong intermolecular hydrogen bonding. The dielectric constant of ammonia (22 at −34 °C [−29 °F]) is lower than that of water (81 at 25 °C [77 °F]), so it is a better solvent for organic materials. However, it is still high enough to allow ammonia to act as a moderately good ionizing solvent. Ammonia also self-ionizes, although less so than does water.
Additional Information
Ammonia is a colorless, poisonous gas with a familiar noxious odor. It occurs in nature, primarily produced by anaerobic decay of plant and animal matter; and it also has been detected in outer space. Some plants, mainly legumes, in combination with rhizobia bacteria, “fix” atmospheric nitrogen to produce ammonia.
Ammonia has been known by its odor since ancient times. It was isolated in the 18th century by notable chemists Joseph Black (Scotland), Peter Woulfe (Ireland), Carl Wilhelm Scheele (Sweden/Germany), and Joseph Priestley (England). In 1785, French chemist Claude Louis Berthollet determined its elemental composition.
Ammonia is produced commercially via the catalytic reaction of nitrogen and hydrogen at high temperature and pressure. The process was developed in 1909 by German chemists Fritz Haber and Carl Bosch. Both received the Nobel Prize in Chemistry for their work, but in widely separated years: Haber in 1918 and Bosch in 1931. The fundamental Haber–Bosch process is still in use today.
In 2020, the worldwide ammonia production capacity was 224 million tonnes (Mt). Actual production was 187 Mt. It ranks ninth among chemicals produced globally.
Most ammonia production—≈85%—is used directly or indirectly in agriculture. Chemical fertilizers made from ammonia include urea, ammonium phosphate, ammonium nitrate, and other nitrates. Other important chemicals produced from ammonia include nitric acid, hydrazine, cyanides, and amino acids.
Ammonia was once used widely as a refrigerant. It has largely been displaced by chlorofluorocarbons and hydrochlorofluorocarbons, which are also under environmental scrutiny. Probably the most familiar household use of ammonia is in glass cleaners.
Ammonia is highly soluble in water; its exact solubility depends on temperature. Aqueous ammonia is also called ammonium hydroxide, but that molecule cannot be isolated. When ammonia is used as a ligand in coordination complexes, it is called “ammine”.
Currently ammonia is made from fossil fuel–derived hydrogen and is therefore not a “green” product, despite its widespread use in agriculture. But environmentally green ammonia may be on the horizon if the hydrogen is made by other means, such as wind- or solar-powered electrolysis of water.
Ammonia can be burned as a fuel in standard engines. A study by the catalyst company Haldor Topsoe (Kongens Lyngby, Denmark) concluded that replacing conventional ship fuels with green ammonia would be cost-efficient and would eliminate a significant source of greenhouse gases. It potentially can be used in aircraft fuels as well. During a transition period, ammonia could be mixed with conventional fuels.

#14 Re: Dark Discussions at Cafe Infinity » crème de la crème » 2025-10-15 18:13:16
2365) Louis Néel
Gist:
Work
Magnetism takes different forms, some stemming from the magnetic moments of atoms of different materials. In ferromagnetic material the magnetic moments are oriented in the same direction. In 1932 Louis Néel described the antiferromagnetism phenomenon, where nearby magnetic moments in a material are oriented in opposite directions. In 1947 he also described the ferrimagnetism phenomenon, where the magnetic moments are aligned in opposite directions but of different magnitudes. The findings became an important factor in the development of computer memory and other applications.
Summary
Louis-Eugène-Félix Néel (born November 22, 1904, Lyon, France—died November 17, 2000, Brive-Corrèze) was a French physicist who was corecipient, with the Swedish astrophysicist Hannes Alfvén, of the Nobel Prize for Physics in 1970 for his pioneering studies of the magnetic properties of solids. His contributions to solid-state physics have found numerous useful applications, particularly in the development of improved computer memory units.
Néel attended the École Normale Supérieure in Paris and the University of Strasbourg (Ph.D., 1932), where he studied under Pierre-Ernest Weiss and first began researching magnetism. He was a professor at the universities of Strasbourg (1937–45) and Grenoble (1945–76), and in 1956 he founded the Center for Nuclear Studies in Grenoble, serving as its director until 1971. Néel also was director (1971–76) of the Polytechnic Institute in Grenoble.
During the early 1930s Néel studied, on the molecular level, forms of magnetism that differ from ferromagnetism. In ferromagnetism, the most common variety of magnetism, the electrons line up (or spin) in the same direction at low temperatures. He discovered that, in some substances, alternating groups of atoms align their electrons in opposite directions (much as when two identical magnets are placed together with opposite poles aligned), thus neutralizing the net magnetic effect. This magnetic property is called antiferromagnetism. Néel’s studies of fine-grain ferromagnetics provided an explanation for the unusual magnetic memory of certain mineral deposits that has provided information on changes in the direction and strength of the Earth’s magnetic field.
Néel wrote more than 200 works on various aspects of magnetism. Mainly because of his contributions, ferromagnetic materials can be manufactured to almost any specifications for technical applications, and a flood of new synthetic ferrite materials has revolutionized microwave electronics.
Details
Louis Eugène Félix Néel (22 November 1904 – 17 November 2000) was a French physicist born in Lyon who received the Nobel Prize for Physics in 1970 for his studies of the magnetic properties of solids.
Biography
Néel studied at the Lycée du Parc in Lyon and was accepted at the École Normale Supérieure in Paris. He obtained the degree of Doctor of Science at the University of Strasbourg. He was corecipient (with the Swedish astrophysicist Hannes Alfvén) of the Nobel Prize for Physics in 1970 for his pioneering studies of the magnetic properties of solids. His contributions to solid state physics have found numerous useful applications, particularly in the development of improved computer memory units. About 1930 he suggested that a new form of magnetic behavior might exist; called antiferromagnetism, as opposed to ferromagnetism. Above a certain temperature (the Néel temperature) this behaviour stops. Néel pointed out (1948) that materials could also exist showing ferrimagnetism. Néel has also given an explanation of the weak magnetism of certain rocks, making possible the study of the history of Earth's magnetic field.
He is the instigator of the Polygone Scientifique in Grenoble.
The Louis Néel Medal, awarded annually by the European Geophysical Society, is named in Néel's honour.
Néel died at Brive-la-Gaillarde on 17 November 2000 at the age 95, just 5 days short of his 96th birthday.

#15 Re: This is Cool » Miscellany » 2025-10-15 17:54:57
2418) Protein
Gist
Protein is a complex molecule made of amino acids that performs vital functions in the body, such as building and repairing tissues, and acting as enzymes and hormones. It is essential for the structure, function, and regulation of the body's tissues and organs. The sequence of amino acids determines a protein's unique three-dimensional shape and specific function.
Protein is important because it serves as the building blocks for your body's cells, helping to build and repair tissues like muscle, bone, and skin. It is essential for numerous bodily functions, including making enzymes and hormones, supporting your immune system, and transporting nutrients.
Summary
Proteins are large biomolecules and macromolecules that comprise one or more long chains of amino acid residues. Proteins perform a vast array of functions within organisms, including catalysing metabolic reactions, DNA replication, responding to stimuli, providing structure to cells and organisms, and transporting molecules from one location to another. Proteins differ from one another primarily in their sequence of amino acids, which is dictated by the nucleotide sequence of their genes, and which usually results in protein folding into a specific 3D structure that determines its activity.
A linear chain of amino acid residues is called a polypeptide. A protein contains at least one long polypeptide. Short polypeptides, containing less than 20–30 residues, are rarely considered to be proteins and are commonly called peptides. The individual amino acid residues are bonded together by peptide bonds and adjacent amino acid residues. The sequence of amino acid residues in a protein is defined by the sequence of a gene, which is encoded in the genetic code. In general, the genetic code specifies 20 standard amino acids; but in certain organisms the genetic code can include selenocysteine and—in certain archaea—pyrrolysine. Shortly after or even during synthesis, the residues in a protein are often chemically modified by post-translational modification, which alters the physical and chemical properties, folding, stability, activity, and ultimately, the function of the proteins. Some proteins have non-peptide groups attached, which can be called prosthetic groups or cofactors. Proteins can work together to achieve a particular function, and they often associate to form stable protein complexes.
Once formed, proteins only exist for a certain period and are then degraded and recycled by the cell's machinery through the process of protein turnover. A protein's lifespan is measured in terms of its half-life and covers a wide range. They can exist for minutes or years with an average lifespan of 1–2 days in mammalian cells. Abnormal or misfolded proteins are degraded more rapidly either due to being targeted for destruction or due to being unstable.
Like other biological macromolecules such as polysaccharides and nucleic acids, proteins are essential parts of organisms and participate in virtually every process within cells. Many proteins are enzymes that catalyse biochemical reactions and are vital to metabolism. Some proteins have structural or mechanical functions, such as actin and myosin in muscle, and the cytoskeleton's scaffolding proteins that maintain cell shape. Other proteins are important in cell signaling, immune responses, cell adhesion, and the cell cycle. In animals, proteins are needed in the diet to provide the essential amino acids that cannot be synthesized. Digestion breaks the proteins down for metabolic use.
Details
Protein is a highly complex substance that is present in all living organisms. Proteins are of great nutritional value and are directly involved in the chemical processes essential for life. The importance of proteins was recognized by chemists in the early 19th century, including Swedish chemist Jöns Jacob Berzelius, who in 1838 coined the term protein, a word derived from the Greek prōteios, meaning “holding first place.” Proteins are species-specific; that is, the proteins of one species differ from those of another species. They are also organ-specific; for instance, within a single organism, muscle proteins differ from those of the brain and liver.
A protein molecule is very large compared with molecules of sugar or salt and consists of many amino acids joined together to form long chains, much as beads are arranged on a string. There are about 20 different amino acids that occur naturally in proteins. Proteins of similar function have similar amino acid composition and sequence. Although it is not yet possible to explain all of the functions of a protein from its amino acid sequence, established correlations between structure and function can be attributed to the properties of the amino acids that compose proteins.
Plants can synthesize all of the amino acids; animals cannot, even though all of them are essential for life. Plants can grow in a medium containing inorganic nutrients that provide nitrogen, potassium, and other substances essential for growth. They utilize the carbon dioxide in the air during the process of photosynthesis to form organic compounds such as carbohydrates. Animals, however, must obtain organic nutrients from outside sources. Because the protein content of most plants is low, very large amounts of plant material are required by animals, such as ruminants (e.g., cows), that eat only plant material to meet their amino acid requirements. Nonruminant animals, including humans, obtain proteins principally from animals and their products—e.g., meat, milk, and eggs. The seeds of legumes are increasingly being used to prepare inexpensive protein-rich food.
The protein content of animal organs is usually much higher than that of the blood plasma. Muscles, for example, contain about 30 percent protein, the liver 20 to 30 percent, and red blood cells 30 percent. Higher percentages of protein are found in hair, bones, and other organs and tissues with a low water content. The quantity of free amino acids and peptides in animals is much smaller than the amount of protein; protein molecules are produced in cells by the stepwise alignment of amino acids and are released into the body fluids only after synthesis is complete.
The high protein content of some organs does not mean that the importance of proteins is related to their amount in an organism or tissue; on the contrary, some of the most important proteins, such as enzymes and hormones, occur in extremely small amounts. The importance of proteins is related principally to their function. All enzymes identified thus far are proteins. Enzymes, which are the catalysts of all metabolic reactions, enable an organism to build up the chemical substances necessary for life—proteins, nucleic acids, carbohydrates, and lipids—to convert them into other substances, and to degrade them. Life without enzymes is not possible. There are several protein hormones with important regulatory functions. In all vertebrates, the respiratory protein hemoglobin acts as oxygen carrier in the blood, transporting oxygen from the lung to body organs and tissues. A large group of structural proteins maintains and protects the structure of the animal body.
Additional Information
Protein is found throughout the body—in muscle, bone, skin, hair, and virtually every other body part or tissue. It makes up the enzymes that power many chemical reactions and the hemoglobin that carries oxygen in your blood. At least 10,000 different proteins make you what you are and keep you that way.
Protein is made from twenty-plus basic building blocks called amino acids. Because we don’t store amino acids, our bodies make them in two different ways: either from scratch, or by modifying others. Nine amino acids—histidine, isoleucine, leucine, lysine, methionine, phenylalanine, threonine, tryptophan, and valine—known as the essential amino acids, must come from food.
The National Academy of Medicine recommends that adults get a minimum of 0.8 grams of protein for every kilogram of body weight per day, or just over 7 grams for every 20 pounds of body weight.
The National Academy of Medicine also sets a wide range for acceptable protein intake—anywhere from 10% to 35% of calories each day. Beyond that, there’s relatively little solid information on the ideal amount of protein in the diet or the healthiest target for calories contributed by protein. Individual needs will vary based on factors such as age, exercise level, health conditions, and overall dietary pattern. A registered dietitian can help determine one’s individual protein needs.
In an analysis conducted at Harvard among more than 130,000 men and women who were followed for up to 32 years, the percentage of calories from total protein intake was not related to overall mortality or to specific causes of death. However, the source of protein was important.
What are “complete” proteins, and how much do I need?
It’s important to note that millions of people worldwide, especially young children, don’t get enough protein due to food insecurity. The effects of protein deficiency and malnutrition range in severity from growth failure and loss of muscle mass to decreased immunity, weakening of the heart and respiratory system, and death.
However, it’s uncommon for healthy adults in the U.S. and most other developed countries to have a deficiency, because there’s an abundance of plant and animal-based foods full of protein. In fact, many in the U.S. are consuming more than enough protein, especially from animal-based foods.

#16 Dark Discussions at Cafe Infinity » Club Quotes - III » 2025-10-15 17:22:52
- Jai Ganesh
- Replies: 0
Club Quotes - III
1. Being at a club that supported me meant a lot. - David Beckham
2. To tell her that I joined the parachute club was too hard for me. I didn't want to trouble her; besides, I was not completely sure about the success of my new adventure. - Valentina Tereshkova
3. A lot of wasted energy in my life has been spent on sorting out problems and issues at Yorkshire cricket. Of course, I know I made mistakes along the way, but I care passionately about the club - I always have done and always will. Geoffrey Boycott
4. At the moment there are some England players who are the stars of their club teams, but not for their country. It's difficult to explain. - Diego Maradona
5. I know I have this level of celebrity, of fame, international, national, whatever you want to call it, but it's a pretty surreal thing to think sometimes that you're in the middle of another famous person's life and you think to yourself, 'How the hell did I get famous? What is this some weird club that we're in?' - Kevin Costner
6. Sugar Breeze, my favourite restaurant in Antigua, serves the best local food, while my local golf club, Cedar Valley, is where I always go for a drink. - Viv Richards
7. At our computer club, we talked about it being a revolution. Computers were going to belong to everyone, and give us power, and free us from the people who owned computers and all that stuff. - Steve Wozniak
8. I decided to create a sports club during the Soviet times. It was my dream. - Sergei Bubka.
#17 Jokes » Autumn Jokes - III » 2025-10-15 17:01:34
- Jai Ganesh
- Replies: 0
Q: How do leaves get from place to place?
A: With autumn-mobiles.
* * *
Q: How does an Elephant get out of a tree?
A: Sits on a leaf and waits till Autumn!
* * *
Q: What did a tree fighting with autumn say?
A: That's it, i'm leaving.
* * *
Q: What will fall on the lawn first?
A: An autumn leaf or a Christmas catalogue.
* * *
Q: What do you call a tree that doubts autumn?
A: Disbe-leaf.
* * *
Q: What is a tree's least favorite month?
A: Sep-timber!
* * *
#18 Re: Jai Ganesh's Puzzles » General Quiz » 2025-10-15 16:13:41
Hi,
#10611. What does the term in Geography Cartouche (cartography) mean?
#10612. What does the term in Geography Waterfall mean?
#19 Re: Jai Ganesh's Puzzles » English language puzzles » 2025-10-15 15:53:13
Hi,
#5807. What does the noun crease mean?
#5808. What does the noun credential mean?
#20 Re: Jai Ganesh's Puzzles » Doc, Doc! » 2025-10-15 15:26:23
Hi,
#2496. What does the medical term Skeletal muscle mean?
#21 Re: Jai Ganesh's Puzzles » 10 second questions » 2025-10-15 14:37:15
Hi,
#9765.
#22 Re: Jai Ganesh's Puzzles » Oral puzzles » 2025-10-15 13:52:02
Hi,
#6271.
#23 Re: Exercises » Compute the solution: » 2025-10-15 13:38:46
Hi,
2615.
#24 Re: This is Cool » Miscellany » 2025-10-14 23:31:40
2417) Locomotive
Gist
A locomotive is a rail vehicle that provides the motive power for a train, either by pulling or pushing it. Powered by sources like electricity or diesel, locomotives have machinery that transmits power to the driving wheels to move the train along the tracks.
A locomotive is a self-propelled, wheeled vehicle that provides the power for a train. It is typically a separate unit that pulls or pushes a train, though the power source can also be incorporated into a car. Modern locomotives are powered by diesel or electricity, which replaced steam as the most common source of power after World War II.
Summary
A locomotive is a rail vehicle that provides the motive power for a train. Traditionally, locomotives pulled trains from the front. However, push–pull operation has become common, and in the pursuit for longer and heavier freight trains, companies are increasingly using distributed power: single or multiple locomotives placed at the front and rear and at intermediate points throughout the train under the control of the leading locomotive.
Etymology
The word locomotive originates from the Latin loco 'from a place', ablative of locus 'place', and the Medieval Latin motivus 'causing motion', and is a shortened form of the term locomotive engine, which was first used in 1814 to distinguish between self-propelled and stationary steam engines.
Classifications
Prior to locomotives, the motive force for railways had been generated by various lower-technology methods such as human power, horse power, gravity or stationary engines that drove cable systems. Few such systems are still in existence today. Locomotives may generate their power from fuel (wood, coal, petroleum or natural gas), or they may take power from an outside source of electricity. It is common to classify locomotives by their source of energy.
Details
A locomotive is any of various self-propelled vehicles used for hauling railroad cars on tracks.
Although motive power for a train-set can be incorporated into a car that also has passenger, baggage, or freight accommodations, it most often is provided by a separate unit, the locomotive, which includes the machinery to generate (or, in the case of an electric locomotive, to convert) power and transmit it to the driving wheels. Today there are two main sources of power for a locomotive: oil (in the form of diesel fuel) and electricity. Steam, the earliest form of propulsion, was in almost universal use until about the time of World War II; since then it has been superseded by the more efficient diesel and electric traction.
The steam locomotive was a self-sufficient unit, carrying its own water supply for generating the steam and coal, oil, or wood for heating the boiler. The diesel locomotive also carries its own fuel supply, but the diesel-engine output cannot be coupled directly to the wheels; instead, a mechanical, electric, or hydraulic transmission must be used. The electric locomotive is not self-sufficient; it picks up current from an overhead wire or a third rail beside the running rails. Third-rail supply is employed only by urban rapid-transit railroads operating on low-voltage direct current.
In the 1950s and ’60s the gas turbine was adopted by one American railroad and some European ones as an alternative to the diesel engine. Although its advantages have been nullified by advances in diesel traction technology and increases in oil price, it is still proposed as an alternative means for installing high-speed rail service for regions where no infrastructure for electric power is in place.
Steam locomotives
The basic features that made George and Robert Stephenson’s Rocket of 1829 successful—its multitube boiler and its system of exhausting the steam and creating a draft in its firebox—continued to be used in the steam locomotive to the end of its career. The number of coupled drive wheels soon increased. The Rocket had only a single pair of driving wheels, but four coupled wheels soon became common, and eventually some locomotives were built with as many as 14 coupled drivers.
Steam-locomotive driving wheels were of various sizes, usually larger for the faster passenger engines. The average was about a 1,829–2,032-mm (72–80-inch) diameter for passenger engines and 1,372–1,676 mm (54–66 inches) for freight or mixed-traffic types.
Supplies of fuel (usually coal but sometimes oil) and water could be carried on the locomotive frame itself (in which case it was called a tank engine) or in a separate vehicle, the tender, coupled to the locomotive. The tender of a typical European main-line locomotive had a capacity of 9,000 kg (10 tons) of coal and 30,000 litres (8,000 gallons) of water. In North America, higher capacities were common.
To meet the special needs of heavy freight traffic in some countries, notably the United States, greater tractive effort was obtained by using two separate engine units under a common boiler. The front engine was articulated, or hinge-connected to the frame of the rear engine, so that the very large locomotive could negotiate curves. The articulated locomotive was originally a Swiss invention, with the first built in 1888. The largest ever built was the Union Pacific’s Big Boy, used in mountain freight service in the western United States. Big Boy weighed more than 600 short tons, including the tender. It could exert 61,400 kg (135,400 pounds) of tractive force and developed more than 6,000 horsepower at 112 km (70 miles) per hour.
One of the best-known articulated designs was the Beyer-Garratt, which had two frames, each having its own driving wheels and cylinders, surmounted by water tanks. Separating the two chassis was another frame carrying the boiler, cab, and fuel supply. This type of locomotive was valuable on lightly laid track; it could also negotiate sharp curves. It was widely used in Africa.
Various refinements gradually improved the reciprocating steam locomotive. Some included higher boiler pressures (up to 2,000–2,060 kilopascals [290–300 pounds per square inch] for some of the last locomotives, compared with about 1,300 kilopascals [200 pounds per square inch] for earlier designs), superheating, feed-water preheating, roller bearings, and the use of poppet (perpendicular) valves rather than sliding piston valves.
Still, the thermal efficiency of even the ultimate steam locomotives seldom exceeded about 6 percent. Incomplete combustion and heat losses from the firebox, boiler, cylinders, and elsewhere dissipated most of the energy of the fuel burned. For this reason the steam locomotive became obsolete, but only slowly, because it had compensating advantages, notably its simplicity and ability to withstand abuse.
Electric traction
Efforts to propel railroad vehicles using batteries date from 1835, but the first successful application of electric traction was in 1879, when an electric locomotive ran at an exhibition in Berlin. The first commercial applications of electric traction were for suburban or metropolitan railroads. One of the earliest came in 1895, when the Baltimore and Ohio electrified a stretch of track in Baltimore to avoid smoke and noise problems in a tunnel. One of the first countries to use electric traction for main-line operations was Italy, where a system was inaugurated as early as 1902.
By World War I a number of electrified lines were operating both in Europe and in the United States. Major electrification programs were undertaken after that war in such countries as Sweden, Switzerland, Norway, Germany, and Austria. By the end of the 1920s nearly every European country had at least a small percentage of electrified track. Electric traction also was introduced in Australia (1919), New Zealand (1923), India (1925), Indonesia (1925), and South Africa (1926). A number of metropolitan terminals and suburban services were electrified between 1900 and 1938 in the United States, and there were a few main-line electrifications. The advent of the diesel locomotive inhibited further trunk route electrification in the United States after 1938, but following World War II such electrification was rapidly extended elsewhere. Today a significant percentage of the standard-gauge track in national railroads around the world is electrified—for example, in Japan (100 percent), Switzerland (92 percent), Belgium (91 percent), the Netherlands (76 percent), Spain (76 percent), Italy (68 percent), Sweden (65 percent), Austria (65 percent), Norway (62 percent), South Korea (55 percent), France (52 percent), Germany (48 percent), China (42 percent), and the United Kingdom (32 percent). By contrast, in the United States, which has some 225,000 km (140,000 miles) of standard-gauge track, electrified routes hardly exist outside the Northeast Corridor, where Amtrak runs the 720-km (450-mile) Acela Express between Boston and Washington, D.C.
The century’s second half also was marked by the creation in cities worldwide of many new electrified urban rapid-transit rail systems, as well as extension of existing systems.
Advantages and disadvantages
Electric traction is generally considered the most economical and efficient means of operating a railroad, provided that cheap electricity is available and that the traffic density justifies the heavy capital cost. Being simply power-converting, rather than power-generating, devices, electric locomotives have several advantages. They can draw on the resources of the central power plant to develop power greatly in excess of their nominal ratings to start a heavy train or to surmount a steep grade at high speed. A typical modern electric locomotive rated at 6,000 horsepower has been observed to develop as much as 10,000 horsepower for a short period under these conditions. Moreover, electric locomotives are quieter in operation than other types and produce no smoke or fumes. Electric locomotives require little time in the shop for maintenance, their maintenance costs are low, and they have a longer life than diesels.
The greatest drawbacks to electrified operation are the high capital investment and maintenance cost of the fixed plant—the traction current wires and structures and power substations—and the costly changes that are usually required in signaling systems to immunize their circuitry against interference from the high traction-current voltages and to adapt their performance to the superior acceleration and sustained speeds obtainable from electric traction.
Types of traction systems
Electric-traction systems can be broadly divided into those using alternating current and those using direct current. With direct current, the most popular line voltages for overhead wire supply systems have been 1,500 and 3,000. Third-rail systems are predominantly in the 600–750-volt range. The disadvantages of direct current are that expensive substations are required at frequent intervals and the overhead wire or third rail must be relatively large and heavy. The low-voltage, series-wound, direct-current motor is well suited to railroad traction, being simple to construct and easy to control. Until the late 20th century it was universally employed in electric and diesel-electric traction units.
The potential advantages of using alternating instead of direct current prompted early experiments and applications of this system. With alternating current, especially with relatively high overhead-wire voltages (10,000 volts or above), fewer substations are required, and the lighter overhead current supply wire that can be used correspondingly reduces the weight of structures needed to support it, to the further benefit of capital costs of electrification. In the early decades of high-voltage alternating current electrification, available alternating-current motors were not suitable for operation with alternating current of the standard commercial or industrial frequencies (50 hertz [cycles per second] in Europe; 60 hertz in the United States and parts of Japan). It was necessary to use a lower frequency (16 2/3 hertz is common in Europe; 25 hertz in the United States); this in turn required either special railroad power plants to generate alternating current at the required frequency or frequency-conversion equipment to change the available commercial frequency into the railroad frequency.
Nevertheless, alternating-current supply systems at 16 2/3 hertz became the standard on several European railroads, such as Austria, Germany, and Switzerland, where electrification began before World War II. Several main-line electrifications in the eastern United States were built using 25-hertz alternating current, which survives in the Northeast Corridor operated by Amtrak.
Interest in using commercial-frequency alternating current in the overhead wire continued, however; and in 1933 experiments were carried out in both Hungary and Germany. The German State Railways electrified its Höllenthal branch at 20,000 volts, 50 hertz.
In 1945 Louis Armand, former president of the French railroads, went ahead with further development of this system and converted a line between Aix-Les-Bains and La Roche-sur-Foron for the first practical experiments. This was so successful that the 25,000-volt, 50- or 60-hertz system has become virtually the standard for new main-line electrification systems.
With commercial-frequency, alternating-current systems, there are two practical ways of taking power to the locomotive driving wheels: (1) by a rotary converter or static rectifier on the locomotive to convert the alternating-current supply into direct current at low voltage to drive standard direct-current traction motors and (2) by a converter system to produce variable-frequency current to drive alternating-current motors. The first method, using nonmechanical rectifiers, was standard practice until the end of the 1970s.
The power-to-weight ratios obtainable with electric traction units had been greatly increased by the end of World War II. Reduction in the bulk of on-board electric apparatus and motors, coupled in the latter with a simultaneous rise in attainable power output, enabled Swiss production for the Bern-Lötschberg-Simplon Railway in 1944 of a 4,000-horsepower locomotive weighing only 80,000 kg (176,370 pounds). Its four axles were all motored. There was no longer need of nonmotorized axles to keep weight on each wheel-set within limits acceptable by the track.
By 1960 the electric industry was producing transformer and rectifier packages slim enough to fit under the frames of a motored urban rapid-transit car, thereby making almost its entire body available for passenger seating. This helped to accelerate and expand the industrialized world’s electrification of metropolitan railway networks for operation by self-powered train-sets (i.e., with some or all vehicles motored). A virtue of the self-powered train-set principle is its easy adaptation to peaks of traffic demand. When two or more sets are coupled, the additional sets have the extra needed traction power. With both electric and diesel traction it is simple to interconnect electrically the power and braking controls of all the train-sets so that the train they form can be driven from a single cab. Because of this facility such train-sets are widely known as multiple-units. Modern multiple-units are increasingly fitted with automatic couplers that combine a draft function with connection of all power, braking, and other control circuits between two train-sets; this is achieved by automatic engagement, when couplers interlock, of a nest of electric contacts built into each coupler head.
From about 1960 major advances in electric traction accrued from the application of electronics. Particularly significant was the perfection of the semiconductor thyristor, or “chopper,” control of current supply to motors. The thyristor—a rapid-action, high-power switch with which the “on” and “off” periods of each cycle can be fractionally varied—achieved smoothly graduated application of voltage to traction motors. Besides eliminating wear-prone parts and greatly improving an electric traction unit’s adhesion, thyristor control also reduced current consumption.
Three-phase alternating-current motor traction became practicable in the 1980s. With electronics it was possible to compress to manageable weight and size the complex equipment needed to transmute the overhead wire or third-rail current to a supply of variable voltage and frequency suitable for feeding to three-phase alternating-current motors. For railroad traction the alternating-current motor is preferable to a direct-current machine on several counts. It is an induction motor with a squirrel-cage rotor (that is, solid conductors in the slots are shorted together by end rings), and it has no commutators or brushes and no mechanically contacting parts except bearings, so that it is much simpler to maintain and more reliable. It is more compact than a direct motor, so more power is obtainable for a specified motor size and weight; the 6,000-kg (14,000-pound) alternating-current motor in each truck of a modern French National Railways electric locomotive delivers a continuous 3,750 horsepower.
The torque of an alternating-current motor increases with speed, whereas that of a direct-current motor is initially high and falls with rising speed; consequently, the alternating-current motor offers superior adhesion for acceleration of heavy trainloads. Finally, the alternating-current motor is more easily switched into a generating mode to act as a dynamic (rheostatic) or regenerative vehicle brake. (In dynamic braking the current generated to oppose the train’s momentum is dissipated through on-board resistances. In regenerative braking, adopted on mountain or intensively operated urban lines where the surplus current can be readily taken up by other trains, it is fed back into the overhead wire or third rail.) The drawbacks of three-phase alternating-current traction are the intricacy of the on-board electrical equipment needed to convert the current supply before it reaches the motors and its higher capital cost by comparison with direct-current motor systems.
A separate traction motor normally serves each axle via a suitably geared drive. For simplicity of final drive it was for many years standard practice to mount the traction motors on a locomotive’s axles. As train speeds rose, it became increasingly important to limit the impact on the track of unsprung masses. Now motors are either suspended within a locomotive’s trucks or, in the case of some high-speed units, suspended from the locomotive’s body and linked to the axles’ final drive gearboxes by flexible drive shafts.
The direct-current motor’s torque:speed characteristics make a locomotive designed for fast passenger trains, whether electric or diesel-electric, generally unsuitable for freight train work. The heavier loads of the latter require different gearing of the final drives—which will reduce maximum speed—and possibly an increase in the number of motored axles, for increased adhesion. But considerable mixed-traffic haulage capability is obtainable with three-phase alternating-current motors because of their superior adhesion characteristics.
Direct-current motor technology was employed in Japan’s first Shinkansen and France’s first Paris-Lyon TGV trains, but by the early 1990s three-phase alternating-current traction had been adopted for both Japanese and European very-high-speed train-sets—and by extension the systems around the world that have been derived from them. In Europe, international train operation without a locomotive change at frontiers is complicated by the railways’ historic adoption of different electrification systems, either 1,500 or 3,000 volts direct current or 25,000 volts 50 hertz or 15,000 volts 16 2/3 hertz alternating current. For instance, TGV-type trains could not operatie at full efficiency between London, Paris, and Brussels on the Eurostar line via the Channel Tunnel as long as they had to accommodate French 25,000-volt alternating-current overhead wire, Belgian 3,000-volt direct-current overhead wire, and British 750-volt third-rail supply. The French had perfected traction units capable of operating on more than one voltage system soon after they decided to adopt 25,000-volt alternating-current electrification in areas not wired at their previous 1,500-volt direct current. Nevertheless, where very-high-speed traction was concerned, it was impossible to contain within acceptable locomotive weight limits the equipment needed for equivalent high-power output under each system. Only after all the new high-speed lines were electrified on high-voltage alternating current was a true high-speed service available on the Eurostar line.
Since about 1980 the performance and economy of both electric and diesel traction units have been considerably advanced by the interposition between driving controls and vital components of microprocessors, which ensure that the components respond with maximum efficiency and that they are not inadvertently overtaxed. Another product of the application of electronics to controls is that in the modern electric locomotive the engine operator can set the train speed he wishes to reach or maintain, and the traction equipment will automatically apply or vary the appropriate power to the motors, taking account of train weight and track gradient. The microprocessors also serve a diagnostic function, continuously monitoring the state of the systems they control for signs of incipient or actual fault. The microprocessors are linked to a main on-board computer that instantly reports the nature and location of an actual or potential malfunction to a visual display in the driving cab, generally with advice for the cab crew on how it might be rectified or its effects temporarily mitigated. The cab display also indicates the effectiveness of the countermeasures taken. The computer automatically stores such data, either for downloading to maintenance staff at the journey’s end or, on a railroad equipped with train-to-ground-installation radio, for immediate transmission to a maintenance establishment so that preparations for repair of a fault are in place as soon as the traction unit ends its run. In newer very-high-speed, fixed-formation train-sets, a through-train fibre-optics transmission system concentrates data from the microprocessor controls—both those of passenger car systems, such as air-conditioning and power-operated entrance doors, and those of the rear locomotive or, in the Japanese Shinkansen train-sets, the traction equipment dispersed among a proportion of its cars.
Diesel traction
By the end of the 1960s, diesel had almost completely superseded steam as the standard railroad motive power on nonelectrified lines around the world. The change came first and most quickly in North America, where, during the 25 years 1935–60 (and especially in the period 1951–60), railroads in the United States completely replaced their steam locomotives.
What caused the diesel to supersede the steam locomotive so rapidly was the pressure of competition from other modes of transport and the continuing rise in wage costs, which forced the railroads to improve their services and adopt every possible measure to increase operating efficiency. Compared with steam, the diesel traction unit had a number of major advantages:
1. It could operate for long periods with no lost time for maintenance; thus, in North America the diesel could operate through on a run of 3,200 km (2,000 miles) or more and then, after servicing, start the return trip. Steam locomotives required extensive servicing after only a few hours’ operation.
2. It used less fuel energy than a steam locomotive, for its thermal efficiency was about four times as great.
3. It could accelerate a train more rapidly and operate at higher sustained speeds with less damage to the track.
In addition, the diesel was superior to the steam locomotive because of its smoother acceleration, greater cleanliness, standardized repair parts, and operating flexibility (a number of diesel units could be combined and run by one operator under multiple-unit control).
The diesel-electric locomotive is, essentially, an electric locomotive that carries its own power plant. Its use, therefore, brings to a railroad some of the advantages of electrification, but without the capital cost of the power distribution and feed-wire system. As compared with an electric locomotive, however, the diesel-electric has an important drawback: since its output is essentially limited to that of its diesel engine, it can develop less horsepower per locomotive unit. Because high horsepower is required for high-speed operation, the diesel is, therefore, less desirable than the electric for high-speed passenger services and very fast freight operations.
Diesel development
Experiments with diesel-engine locomotives and railcars began almost as soon as the diesel engine was patented by the German engineer Rudolf Diesel in 1892. Attempts at building practical locomotives and railcars (for branch-line passenger runs) continued through the 1920s. The first successful diesel switch engine went into service in 1925; “road” locomotives were delivered to the Canadian National and New York Central railroads in 1928. The first really striking results with diesel traction were obtained in Germany in 1933. There, the Fliegende Hamburger, a two-car, streamlined, diesel-electric train, with two 400-horsepower engines, began running between Berlin and Hamburg on a schedule that averaged 124 km (77 miles) per hour. By 1939 most of Germany’s principal cities were interconnected by trains of this kind, scheduled to run at average speeds up to 134.1 km (83.3 miles) per hour between stops.
The next step was to build a separate diesel-electric locomotive unit that could haul any train. In 1935 one such unit was delivered to the Baltimore and Ohio and two to the Santa Fe Railway Company. These were passenger units; the first road freight locomotive, a four-unit, 5,400-horsepower Electro-Motive Division, General Motors Corporation demonstrator, was not built until 1939.
By the end of World War II, the diesel locomotive had become a proven, standardized type of motive power, and it rapidly began to supersede the steam locomotive in North America. In the United States a fleet of 27,000 diesel locomotives proved fully capable of performing more transportation work than the 40,000 steam locomotives they replaced.
After World War II, the use of diesel traction greatly increased throughout the world, though the pace of conversion was generally slower than in the United States.
Elements of the diesel locomotive
Although the diesel engine has been vastly improved in power and performance, the basic principles remain the same: drawing air into the cylinder, compressing it so that its temperature is raised, and then injecting a small quantity of oil into the cylinder. The oil ignites without a spark because of the high temperature. The diesel engine may operate on the two-stroke or four-stroke cycle. Rated operating speeds vary from 350 to 2,000 revolutions per minute, and rated output may be from 10 to 4,000 horsepower. Railroads in the United States use engines in the 1,000-revolutions-per-minute range; in Europe and elsewhere, some manufacturers have favoured more compact engines of 1,500–2,000 revolutions per minute.
Most yard-switching and short-haul locomotives are equipped with diesel engines ranging from 600 to 1,800 horsepower; road units commonly have engines ranging from 2,000 to 4,000 horsepower. Most builders use V-type engines, although in-line types are used on smaller locomotives and for underfloor fitment on railcars and multiple-unit train-sets.
The most commonly employed method of power transmission is electric, to convert the mechanical energy produced by the diesel engine to current for electric traction motors. Through most of the 20th century the universal method was to couple the diesel engine to a direct-current generator, from which, through appropriate controls, the current was fed to the motors. Beginning in the 1970s, the availability of compact semiconductor rectifiers enabled replacement of the direct-current generator by an alternator, which is able to produce more power and is less costly to maintain than an equivalent direct-current machine. For supply of series-wound direct-current traction motors, static rectifiers converted the three-phase alternating-current output of the alternator to direct current. Then in the 1980s European manufacturers began to adopt the three-phase alternating-current motor for diesel-electric traction units seeking advantages similar to those obtainable from this technology in electric traction. This requires the direct-current output from the rectifier to be transmuted by a thyristor-controlled inverter into a three-phase variable voltage and frequency supply for the alternating-current motors.
On some railroads with lightly laid track, generally those with narrow rail gauge, locomotives may still need nonmotored as well as motored axles for acceptable weight and bulk distribution. But the great majority of diesel-electric locomotives now have all axles powered.
Other types of transmissions also are used in diesel locomotives. The hydraulic transmission, which first became quite popular in Germany, is often favoured for diesel railcars and multiple-unit train-sets. It employs a centrifugal pump or impeller driving a turbine in a chamber filled with oil or a similar fluid. The pump, driven by the diesel engine, converts the engine power to kinetic energy in the oil impinging on the turbine blades. The faster the blades move, the less the relative impinging speed of the oil and the faster the locomotive moves.
Mechanical transmission is the simplest type; it is mainly used in very low-power switching locomotives and in low-power diesel railcars. Basically it is a clutch and gearbox similar to those used in automobiles. A hydraulic coupling, in some cases, is used in place of a friction clutch.
Types of diesel motive power
There are three broad classes of railroad equipment that use diesel engines as prime movers:
1. The light passenger railcar or rail bus (up to 200 horsepower), which usually is four-wheeled and has mechanical transmission. It may be designed to haul a light trailer car. Use of such vehicles is very limited.
2. The four-axle passenger railcar (up to 750 horsepower), which can be operated independently, haul a nonpowered trailer, or be formed into a semipermanent train-set such as a multiple-unit with all or a proportion of the cars powered. In the powered cars the diesel engine and all associated traction equipment, including fuel tanks, are capable of fitting under the floor to free space above the frames for passenger seating. Transmission is either electric or hydraulic. Modern railcars and railcar train-sets are mostly equipped for multiple-unit train operation, with driving control from a single cab.
3. Locomotives (10 to 4,000 horsepower), which may have mechanical transmission if very low-powered or hydraulic transmission for outputs of up to about 2,000 horsepower but in most cases have electric transmission, the choice depending on power output and purpose.
A substantial increase of diesel engine power-to-weight ratios and the application of electronics to component control and diagnostic systems brought significant advances in the efficiency of diesel locomotives in the last quarter of the 20th century. In 1990 a diesel engine with a continuous rating of 3,500 horsepower was available at almost half the weight of a similar model in 1970. At the same time, the fuel efficiency of diesel engines was significantly improved.
Electronics have made a particularly important contribution to the load-hauling capability of diesel-electric locomotives in road freight work, by improving adhesion at starting or in grade-climbing. A locomotive accelerating from rest can develop from 33 to 50 percent more tractive force if its powered wheels are allowed to “creep” into a very slight, steady, and finely controlled slip. In a typical “creep control” system, Doppler radar mounted under the locomotive precisely measures true ground speed, against which microprocessors calculate the ideal creep speed limit in the prevailing track conditions and automatically regulate current supply to the traction motors. The process is continuous, so that current levels are immediately adjusted to match a change in track parameters. In the 1960s, North Americans considered that a diesel-electric locomotive of 3,000–3,600 horsepower or more must have six motored axles for effective adhesion: two railroads had acquired a small number of eight-motored-axle locomotives, each powered by two diesel engines, with outputs of 5,000–6,600 horsepower. Since the mid-1980s four-axle locomotives of up to 4,000 horsepower have become feasible and are widely employed in fast freight service (though for heavy freight duty six-axle locomotives were still preferred). But today a 4,000-horsepower rating is obtainable from a 16-cylinder diesel engine, whereas in the 1960s a 3,600-horsepower output demanded a 20-cylinder engine. This, coupled with the reduction in the number of locomotives required to haul a given tonnage due to improved adhesion, has been a key factor in decreasing locomotive maintenance costs.
Outside North America, widespread electrification all but ended production of diesel locomotives purpose-built for passenger train haulage in the 1960s. The last development for high-speed diesel service was on British Railways, which, for its nonelectrified trunk routes, mass-produced a semipermanent train-set, the InterCity 125, that had a 2,250-horsepower locomotive at each end of seven or eight intermediate cars. In 1987 one of these sets established a world speed record for diesel traction of 238 km (148 miles) per hour. Some InterCity 125 sets are expected to remain in service under various other designations until well into the 21st century. In North America, Amtrak in the United States and VIA in Canada, as well as some urban mass-transit authorities, still operate diesel locomotives exclusively on passenger trains. Elsewhere road haul diesel locomotives are designed either for exclusive freight haulage or for mixed passenger and freight work.
Traction operating methods
Multiple-unit connection and operation of locomotives, to adjust power to load and track gradient requirements, is standard practice in North America and is common elsewhere. Where considerable gradients occur or freight trains are unusually long and heavy, concentration of locomotives at a train’s head can strain couplings and undesirably delay transmission of full braking power to the train’s rearmost cars. In such conditions several railroads, principally in North America, employ crewless “slave” locomotives that are inserted partway down the train. Radio signals transmitted from the train’s leading locomotive cause the slave locomotive’s controls to respond automatically and correspondingly to all operations of the controls. A world record for freight train weight and length was set in August 1989 on South Africa’s electrified, 830-km (516-mile), 1,065-mm (3-foot 6-inch) gauge Sishen-Saldanha ore line. In the course of research into the feasibility of increasing the line’s regular trainloads, a 660-car train grossing 71,600 tons and 7.2 km (4.47 miles) long was run from end to end of the route. Power was furnished by five 5,025-horsepower electric locomotives at the front, four more inserted after the 470th freight car, and at the rear, to avoid overtaxing the traction current supply system, seven 2,900-horsepower diesel locomotives.
After World War II easy directional reversibility of passenger train-sets became increasingly important for intensively operated short- and medium-haul services, to reduce terminal turnround times and minimize the number of train-sets needed to provide the service. The most popular medium has been the self-powered railcar or multiple-unit train-set, with a driving cab at each end, so that reversal requires only that the crew change cabs. An alternative, known as push-pull, has a normal locomotive at one end and, at the other, a nonpowered passenger or baggage car, known as the driving or control trailer, with a driving cab at its extremity. In one direction the locomotive pulls the train; in the other, unmanned, it propels the train, driven via through-train wiring from the control trailer’s cab. A potential operating advantage of push-pull as opposed to use of self-powered train-sets on a railroad running both passenger and freight trains is that at night, when passenger operation has ceased, the locomotives can be detached for freight haulage.
Turbine propulsion
In the 1950s gas-turbine instead of diesel propulsion was tried for a few locomotives in the United States and Britain, but the results did not justify continuing development. There was a longer but very limited career in rail use for the compact and lightweight gas turbines developed for helicopters that became available in the 1960s. Their power-to-weight ratio, superior to that of contemporary diesel engines, made them preferable for lightweight, high-speed train-sets. They were applied to Canadian-built train-sets placed in service in 1968 between Montreal and Toronto and in 1969 between New York City and Boston, but these were short-lived because of equipment troubles, operating noise, and the cost of fuel. The technology has not been entirely abandoned, however. At the end of the 20th and beginning of the 21st centuries, the Bombardier company of Canada presented its gas-turbine JetTrain locomotive as an alternative to electric traction for new North American high-speed systems.
Several attempts have been made to adapt the steam turbine to railroad traction. One of the first such experiments was a Swedish locomotive built in 1921. Other prototypes followed in Europe and the United States. They all functioned, but they made their appearance too late to compete against the diesel and electrification.

#25 Dark Discussions at Cafe Infinity » Club Quotes - II » 2025-10-14 21:56:11
- Jai Ganesh
- Replies: 0
Club Quotes - II
1. I can remember getting rejected systematically by casting directors as a young kid. I felt like the biggest outsider there ever was; that I'd never belong in that club. - Leonardo DiCaprio
2. I have heard good things about Somerset from Azhar Ali, and I want to play a part in the Club winning matches. - Babar Azam
3. I've never been much of a computer guy at least in terms of playing with computers. Actually until I was about 11 I didn't use a computer for preparing for games at all. I was playing a bit online, was using the chess club mainly. Now, obviously, the computer is an important tool for me preparing for my games. - Magnus Carlsen
4. I'm proud to play for Real Madrid because I have fun; when you no longer have fun it's a sign that it's time to leave. For now though, I'm happy here at the greatest club in the world. - Cristiano Ronaldo
5. A perfectly straight shot with a big club is a fluke. - Jack Nicklaus
6. It's time to stop thinking of the Republican Party as an exclusive club where your ideological card is checked at the door, and start thinking about how we can attract more solution-based leaders like Nathan Fletcher and Anthony Adams. - Arnold Schwarzenegger
7. When I was 7, I started playing with a club. The only grass on the field was in the corner. There was no grass in the middle! It was just sand. - Ronaldinho
8. Every once in a while, we'd ask my dad if we could get a ride in one of these planes. And, he did take us to the flying club and get us a ride in the Pushpak and a glider that the flying club had. - Kalpana Chawla.