Math Is Fun Forum
You are not logged in.
- Topics: Active | Unanswered
Pages: 1
#1 2006-01-12 08:24:20
- mikau
- Member

- Registered: 2005-08-22
- Posts: 1,504
Real life architectual problem
This was a real architectual problem my dad encountered, and it took him forever to solve. Lets see if anyone can get it:
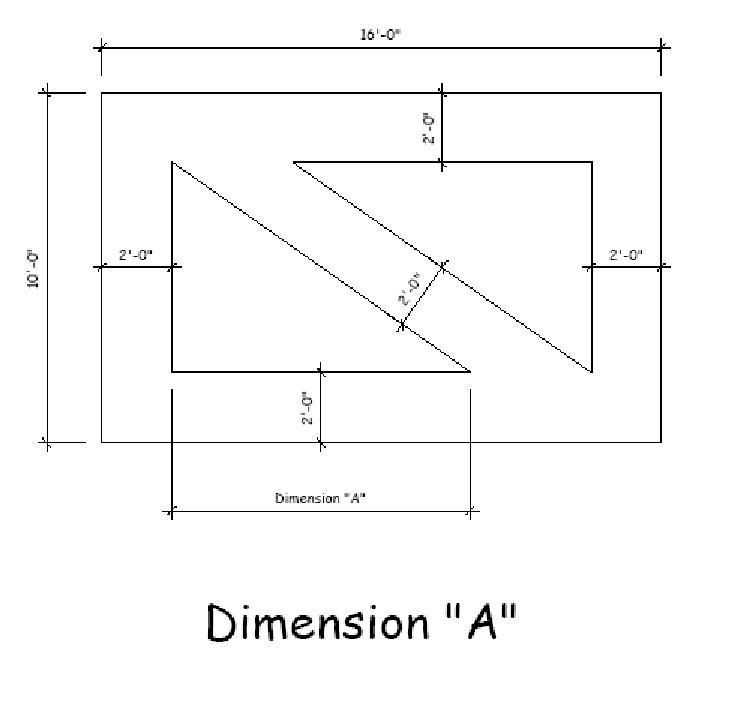
Find A.
Last edited by mikau (2006-01-12 08:24:40)
A logarithm is just a misspelled algorithm.
Offline
#2 2006-01-12 11:14:34
- MathsIsFun
- Administrator

- Registered: 2005-01-21
- Posts: 7,713
Re: Real life architectual problem
Nice puzzle ... could be a worksheet ![]()
"The physicists defer only to mathematicians, and the mathematicians defer only to God ..." - Leon M. Lederman
Offline
#3 2006-01-12 12:15:25
- mikau
- Member

- Registered: 2005-08-22
- Posts: 1,504
Re: Real life architectual problem
I don't even remember how to solve it. :-P
A logarithm is just a misspelled algorithm.
Offline
#4 2006-01-12 17:18:03
- MathsIsFun
- Administrator

- Registered: 2005-01-21
- Posts: 7,713
Re: Real life architectual problem
Step 1: reduce bounding rectangle by 2 × 2', to become 6' by 12'
"The physicists defer only to mathematicians, and the mathematicians defer only to God ..." - Leon M. Lederman
Offline
#5 2006-01-12 17:24:59
- mikau
- Member

- Registered: 2005-08-22
- Posts: 1,504
Re: Real life architectual problem
Step 2. Eat a banana, and think what to do in step 3.
A logarithm is just a misspelled algorithm.
Offline
#6 2006-01-12 18:10:04
- Ricky
- Moderator

- Registered: 2005-12-04
- Posts: 3,791
Re: Real life architectual problem
Triangle on the left is triangle A, triangle on the right is triangle B. Angle x is at the top of triangle A and angle y is at the bottom right of triangle A. I have to assume both triangles are similar, so angle x is also at the bottom of triangle B and angle y is at the top left of triangle B.
Draw a line from the point of angle y in triangle B to the hypotenuse of triangle A, such that it hits the hypotenuse at a 90 degree angle. Then, draw a line which connects the very top of triangle A to that of B. Call this new triangle, triangle C.
The bottom most angle of triangle C is 90 degrees. Since the hypotenuses in triangles A and B are parallel (assumed), the top left angle in triangle C is equal to angle y in triangle B. Now add the top right angle of triangle C, the 90 degree angle just to the right of it, and angle y in triangle B. Since all these angles put together form a line, they add up to 180 degrees. But we also know that x + y + 90 = 180. Thus, the top right angle in C is equal to angle x. Therefore, triangle C is similar to triangle B and A (assuming these two are congruent).
However, we know that the side of triangle C is 2'. Since the triangles are similar: 6 / 12 = 2 / x, 6x = 24, x = 4. So the other side of triangle C is 4'. This means the hypotenuse of triangle C is about 4.47'.
The length of the entire bottom is 12 feet, 12 - 4.47 = 7.53'.
Last edited by Ricky (2006-01-12 18:17:49)
"In the real world, this would be a problem. But in mathematics, we can just define a place where this problem doesn't exist. So we'll go ahead and do that now..."
Offline
#7 2006-01-12 18:44:52
- mikau
- Member

- Registered: 2005-08-22
- Posts: 1,504
Re: Real life architectual problem
Forgive me if I'm wrong but I think you messed up. It apppears you scalled the inside triangle to a 12 by 6 triangle. You can't, they are not congruent:
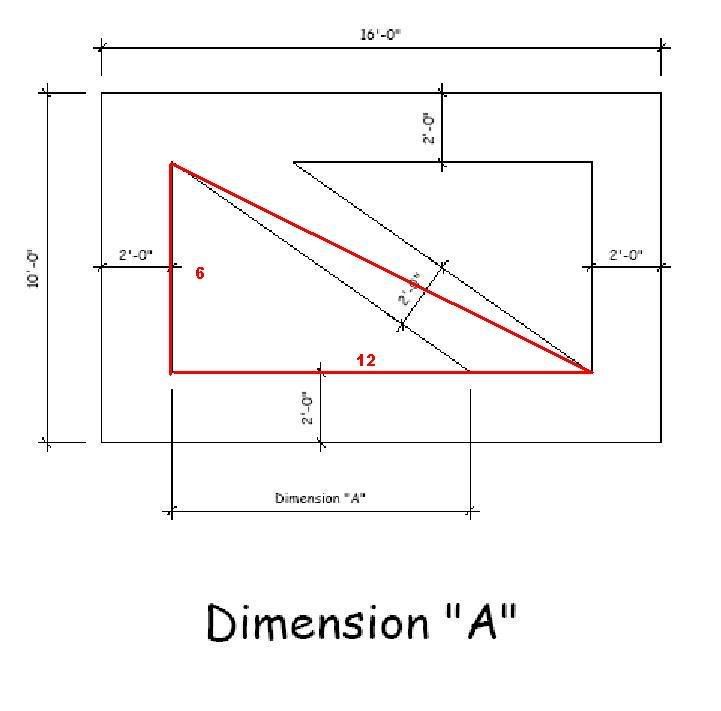
A logarithm is just a misspelled algorithm.
Offline
#8 2006-01-12 18:49:32
- Ricky
- Moderator

- Registered: 2005-12-04
- Posts: 3,791
Re: Real life architectual problem
![]()
Then I give up. I'm not quite sure if it is possible since you don't know any of the angles.
Of course, if your father measured one single angle, he would have saved me 30 minutes of work. ![]()
"In the real world, this would be a problem. But in mathematics, we can just define a place where this problem doesn't exist. So we'll go ahead and do that now..."
Offline
#9 2006-01-12 18:54:59
- mikau
- Member

- Registered: 2005-08-22
- Posts: 1,504
Re: Real life architectual problem
Lol. Theres actually a trick to it. And a hint.
The hint is litterally this: "think outside the box".
A logarithm is just a misspelled algorithm.
Offline
#10 2006-01-12 19:06:32
- Ricky
- Moderator

- Registered: 2005-12-04
- Posts: 3,791
Re: Real life architectual problem
Ok, here's me thinking outside the box.
Take a protractor, measure the angle of the triangle, use this and tan to find A.
Problem solved.
"In the real world, this would be a problem. But in mathematics, we can just define a place where this problem doesn't exist. So we'll go ahead and do that now..."
Offline
#11 2006-01-12 19:09:28
- mikau
- Member

- Registered: 2005-08-22
- Posts: 1,504
Re: Real life architectual problem
This is achictecture, you can't measure it untill its either built or drawn, which requires you know the dimensions. (evil laughter)
A logarithm is just a misspelled algorithm.
Offline
#12 2006-01-12 21:04:17
- MathsIsFun
- Administrator

- Registered: 2005-01-21
- Posts: 7,713
Re: Real life architectual problem
I am confused ... are you saying the two triangles are not congruent?
If they are congruent, then we need to find the gap "B" (=12-A)
And the gap "B" will be a function of the angle θ
And θ will be a function of "B"
Round and round we go ... ![]()
"The physicists defer only to mathematicians, and the mathematicians defer only to God ..." - Leon M. Lederman
Offline
#13 2006-01-13 03:20:54
- mikau
- Member

- Registered: 2005-08-22
- Posts: 1,504
Re: Real life architectual problem
The two triangles are congruent to eachother. But they are obviously not congruent to the red triangle I drew. Ricky incorrectly scaled from this triangle.
A logarithm is just a misspelled algorithm.
Offline
#14 2006-01-13 10:00:27
- MathsIsFun
- Administrator

- Registered: 2005-01-21
- Posts: 7,713
Re: Real life architectual problem
OK, how about
"The physicists defer only to mathematicians, and the mathematicians defer only to God ..." - Leon M. Lederman
Offline
#15 2006-01-13 11:04:45
- mikau
- Member

- Registered: 2005-08-22
- Posts: 1,504
Re: Real life architectual problem
(long low whistle) That is utterly genius! And may be an entirely different way of solving the problem!
Last edited by mikau (2006-01-13 11:06:38)
A logarithm is just a misspelled algorithm.
Offline
#16 2006-01-13 17:32:12
- mikau
- Member

- Registered: 2005-08-22
- Posts: 1,504
Re: Real life architectual problem
Well I"ve been thinking about it, but unless I'm mistaken, it appears that only produces another unknown dimension, so that doesn't help.
I shall reveal the solution to the problem momentarily.
A logarithm is just a misspelled algorithm.
Offline
#17 2006-01-13 18:06:45
- mikau
- Member

- Registered: 2005-08-22
- Posts: 1,504
Re: Real life architectual problem
Here we are! The solution to this nightmare of a problem is really quite simple, it just requires a little trick.
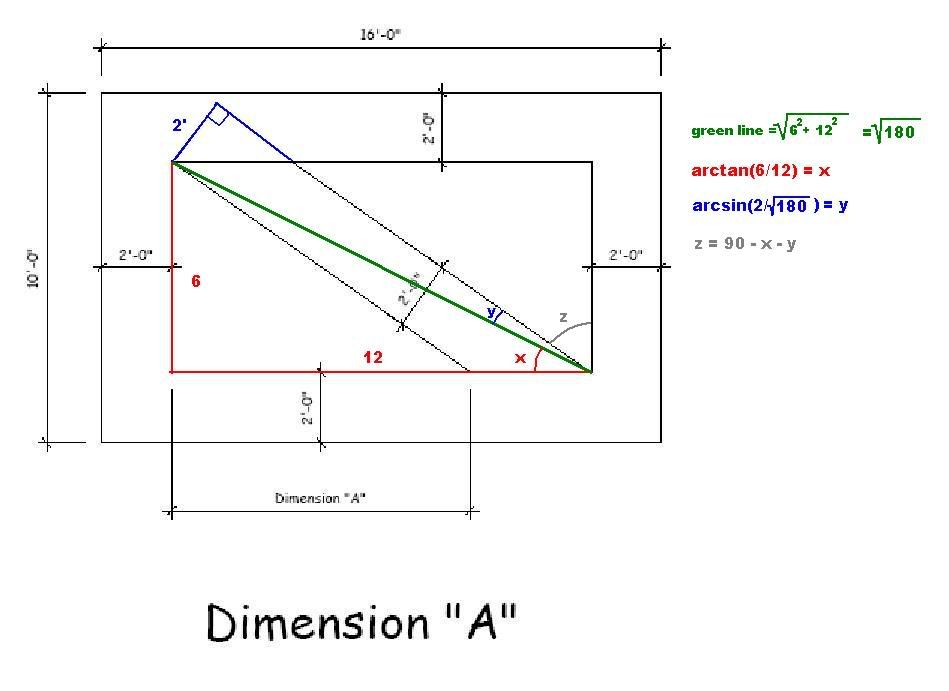
As you can see, the trick is to extend the beam out just enough till it touches the uper left corner and the required lengths and angles can be found as shown. Once you have these, the solution to the problem is easily attainable.
A logarithm is just a misspelled algorithm.
Offline
#18 2006-01-13 20:43:59
- MathsIsFun
- Administrator

- Registered: 2005-01-21
- Posts: 7,713
Re: Real life architectual problem
You are right, my new method is not as good as it looks ![]()
But I can't help but think that a there may be some obvious way to lay it out.
I know - give the job to a carpenter!
Let me see ... they would mark lines 2' in from the outer border, and form a rectangle. Then they would probably draw a circle around one corner 2' in radius, then put a nail in the other corner, attach a string, and run the string so it was tangent to the circle at the first corner! ![]()
So, it would look a bit like your solution, then!
"The physicists defer only to mathematicians, and the mathematicians defer only to God ..." - Leon M. Lederman
Offline
#19 2006-01-14 11:10:26
- mikau
- Member

- Registered: 2005-08-22
- Posts: 1,504
Re: Real life architectual problem
Thats a good way to do it. :-)
A logarithm is just a misspelled algorithm.
Offline
Pages: 1