Math Is Fun Forum
You are not logged in.
- Topics: Active | Unanswered
#26 2007-11-07 04:26:29
- mathsyperson
- Moderator

- Registered: 2005-06-22
- Posts: 4,900
Re: Chess Puzzles
Why did the vector cross the road?
It wanted to be normal.
Offline
#27 2007-11-07 04:47:26
- JaneFairfax
- Member

- Registered: 2007-02-23
- Posts: 6,868
Re: Chess Puzzles
Offline
#28 2007-11-07 21:39:05
- JohnnyReinB
- Member

- Registered: 2007-10-08
- Posts: 453
Re: Chess Puzzles
"There is not a difference between an in-law and an outlaw, except maybe that an outlaw is wanted" ![]()
Nisi Quam Primum, Nequequam
Offline
#29 2007-11-08 17:58:16
- Jai Ganesh
- Administrator

- Registered: 2005-06-28
- Posts: 52,223
Re: Chess Puzzles
Solution to #15, #16 and #17.
mathsyperson, JaneFairfax, and JohnnyReinB! All the answers are correct!
Outstanding!
It appears you three are seasoned players ![]()
PS:- I am sorry for posting the same question 15 1nd 16. By oversight!
To mathsy : It can be forced on the defending King.
#18. Can a king and Bishop alone checkamte the other King who has no powers or pawns and is alone on the Board?
#18 B : Can a King and a Bishop, with a pawn do the same as in #18?
DIFIICULTY LEVEL : 2
It appears to me that if one wants to make progress in mathematics, one should study the masters and not the pupils. - Niels Henrik Abel.
Nothing is better than reading and gaining more and more knowledge - Stephen William Hawking.
Offline
#30 2007-11-09 00:47:01
- Identity
- Member

- Registered: 2007-04-18
- Posts: 934
Re: Chess Puzzles
Offline
#31 2007-11-09 01:49:00
- Jai Ganesh
- Administrator

- Registered: 2005-06-28
- Posts: 52,223
Re: Chess Puzzles
Solutions to #18 and #18B:
Very good! Well done, Identity!!!
It appears to me that if one wants to make progress in mathematics, one should study the masters and not the pupils. - Niels Henrik Abel.
Nothing is better than reading and gaining more and more knowledge - Stephen William Hawking.
Offline
#32 2007-11-18 22:47:52
- JohnnyReinB
- Member

- Registered: 2007-10-08
- Posts: 453
Re: Chess Puzzles
I have a different answer for
Last edited by JohnnyReinB (2007-11-18 22:48:20)
"There is not a difference between an in-law and an outlaw, except maybe that an outlaw is wanted" ![]()
Nisi Quam Primum, Nequequam
Offline
#33 2007-11-19 01:08:54
- JaneFairfax
- Member

- Registered: 2007-02-23
- Posts: 6,868
Re: Chess Puzzles
Last edited by JaneFairfax (2007-11-19 01:12:37)
Offline
#34 2007-11-20 21:29:59
- JohnnyReinB
- Member

- Registered: 2007-10-08
- Posts: 453
Re: Chess Puzzles
Ok, it has to be in opposition. Thanks for the correction, Jane
"There is not a difference between an in-law and an outlaw, except maybe that an outlaw is wanted" ![]()
Nisi Quam Primum, Nequequam
Offline
#35 2007-11-23 23:28:37
- JohnnyReinB
- Member

- Registered: 2007-10-08
- Posts: 453
Re: Chess Puzzles
It has occurred to me that Ganesh seems to be missing these last few days.
Does anyone have any ideas to why this is so?
"There is not a difference between an in-law and an outlaw, except maybe that an outlaw is wanted" ![]()
Nisi Quam Primum, Nequequam
Offline
#36 2007-12-04 00:16:04
- Jai Ganesh
- Administrator

- Registered: 2005-06-28
- Posts: 52,223
Re: Chess Puzzles
JohnyReinB,
Gnaesh is back, alive and kicking!!!!
Don't worry!!!!
Thanks, JaneFaiefarfax, for that beautiful picture!
It appears to me that if one wants to make progress in mathematics, one should study the masters and not the pupils. - Niels Henrik Abel.
Nothing is better than reading and gaining more and more knowledge - Stephen William Hawking.
Offline
#37 2007-12-11 20:05:53
- Jai Ganesh
- Administrator

- Registered: 2005-06-28
- Posts: 52,223
Re: Chess Puzzles
#19. White King and white Queen in their starting position. Black King in starting position. Rest of the board empty. In minimum how many moves can white checkmate black? White to play.
#20. White King and Queen side Rook in starting position. Black King in starting position. Rest of the Board empty. In how many moves (minimum) can White checkmate Black?
It appears to me that if one wants to make progress in mathematics, one should study the masters and not the pupils. - Niels Henrik Abel.
Nothing is better than reading and gaining more and more knowledge - Stephen William Hawking.
Offline
#38 2007-12-12 02:08:14
- mathsyperson
- Moderator

- Registered: 2005-06-22
- Posts: 4,900
Re: Chess Puzzles
Is Black being co-operative?
Why did the vector cross the road?
It wanted to be normal.
Offline
#39 2007-12-12 02:09:43
- Jai Ganesh
- Administrator

- Registered: 2005-06-28
- Posts: 52,223
Re: Chess Puzzles
Not at all! It has to be forced on Black!!!
It appears to me that if one wants to make progress in mathematics, one should study the masters and not the pupils. - Niels Henrik Abel.
Nothing is better than reading and gaining more and more knowledge - Stephen William Hawking.
Offline
#40 2007-12-12 08:18:30
- JaneFairfax
- Member

- Registered: 2007-02-23
- Posts: 6,868
Re: Chess Puzzles
Offline
#41 2007-12-12 16:56:09
- Jai Ganesh
- Administrator

- Registered: 2005-06-28
- Posts: 52,223
Re: Chess Puzzles
Excellent, solutions are correct, JaneFairfax!!!
It appears to me that if one wants to make progress in mathematics, one should study the masters and not the pupils. - Niels Henrik Abel.
Nothing is better than reading and gaining more and more knowledge - Stephen William Hawking.
Offline
#42 2007-12-12 22:38:35
- JaneFairfax
- Member

- Registered: 2007-02-23
- Posts: 6,868
Re: Chess Puzzles
#21. White to move and force mate in two moves.
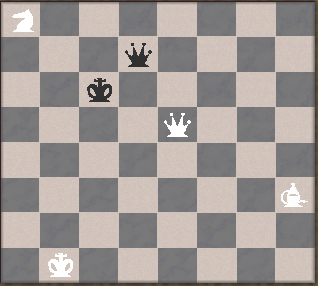
Simple problem I made up myself (difficulty level: 4). ![]()
Offline
#43 2007-12-12 22:46:40
- Jai Ganesh
- Administrator

- Registered: 2005-06-28
- Posts: 52,223
Re: Chess Puzzles
1. Bg2 Check - Qd4
2. QxQ Checkmate
It appears to me that if one wants to make progress in mathematics, one should study the masters and not the pupils. - Niels Henrik Abel.
Nothing is better than reading and gaining more and more knowledge - Stephen William Hawking.
Offline
#44 2007-12-12 23:26:29
- NullRoot
- Member

- Registered: 2007-11-19
- Posts: 162
Re: Chess Puzzles
Nice puzzle. It's so tempting to take that queen out with the bishop ![]() .
.
Last edited by NullRoot (2007-12-12 23:26:49)
Trillian: Five to one against and falling. Four to one against and falling Three to one, two, one. Probability factor of one to one. We have normality. I repeat, we have normality. Anything you still cant cope with is therefore your own problem.
Offline
#45 2007-12-13 10:43:29
- JaneFairfax
- Member

- Registered: 2007-02-23
- Posts: 6,868
Re: Chess Puzzles
Excellent job, Ganesh and NullRoot! Bravo. ![]()
Fancy another one? Heres another one I made up myself. ![]()
#22. As before, White to play and mate in two moves. This one is a bit trickier (difficulty level: 6) so good luck. ![]()
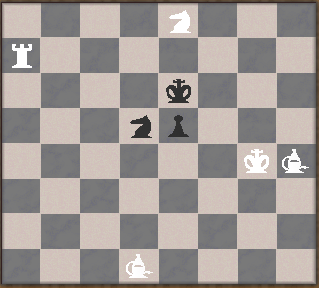
Oh, and using the [hide] tag would be a good idea. ![]()
Offline
#46 2007-12-13 11:36:15
- NullRoot
- Member

- Registered: 2007-11-19
- Posts: 162
Re: Chess Puzzles
Nice ![]()
Trillian: Five to one against and falling. Four to one against and falling Three to one, two, one. Probability factor of one to one. We have normality. I repeat, we have normality. Anything you still cant cope with is therefore your own problem.
Offline
#47 2007-12-13 11:37:19
- mathsyperson
- Moderator

- Registered: 2005-06-22
- Posts: 4,900
Re: Chess Puzzles
Grr...
Why did the vector cross the road?
It wanted to be normal.
Offline
#48 2007-12-13 12:05:06
- JaneFairfax
- Member

- Registered: 2007-02-23
- Posts: 6,868
Re: Chess Puzzles
Excellent work! Wow, you guys rock! ![]()
Thats all the chess puzzles I have so far. Gotta think of some more (and make em much harder ones), I guess. ![]()
Offline
#49 2007-12-13 13:44:56
- mathsyperson
- Moderator

- Registered: 2005-06-22
- Posts: 4,900
Re: Chess Puzzles
What do you use to make those Chessy images, Jane?
Why did the vector cross the road?
It wanted to be normal.
Offline
#50 2007-12-13 21:21:23
- NullRoot
- Member

- Registered: 2007-11-19
- Posts: 162
Re: Chess Puzzles
I really need to get back into chess. I think I'll have to learn all over again though!
Trillian: Five to one against and falling. Four to one against and falling Three to one, two, one. Probability factor of one to one. We have normality. I repeat, we have normality. Anything you still cant cope with is therefore your own problem.
Offline