Math Is Fun Forum

katy

Member

Registered: 2005-12-28

Posts: 14

Circular Functions!!!

I am stuck with this one...can anyone please show me how to do this step by step please...

The proplem is:
sin 2x=1/2

and the answers are:
2x=30degrees,150degrees,390degrees,410degrees
  x=15degrees,75degrees,195degrees,205degrees

how do you know to do this??...

Last edited by katy (2006-03-05 08:48:41)

katy

Member

Registered: 2005-12-28

Posts: 14

Re: Circular Functions!!!

help me please...this is urgent guys...huhuhu

mikau

Member

Registered: 2005-08-22

Posts: 1,504

Re: Circular Functions!!!

ok theres the frequently discussed 30, 60, 90 degree triangle. You remember that. This shows us that the sine of 30 = 1/2. Also the sine of 150 = 1/2, and of course, you can always add increments of 360 to get the same sine.

Ok, so the sine of 30 = 1/2   the sine of 150 = 1/2   if the sin (2x) = 1/2 then substituting we get sin (2x) = sin 30 and sin (2x) = sin(150)       therefore its obvious that 2x equals either 150 or 30, 2x = 30 or 2x = 150 so x = 15 or 75.

But also sin (390) = 1/2,  and sin (410) = 1/2 since 390 = 30 degree's and 410 = 150 degree's (we just looped around an extra time)

so 2x = 390 or 2x = 410  solving we get x = 195, or x = 205.

Why did we bother to add 360 to 30 and 150? Adding 360 degree's to an angle will not change its direction at all,  so the sines will still be the same as that of the original angle. The point is sine (2x) = 1/2, so we want to know all values between 0 and 360 where sin (2x) = 1/2. If we'd stopped at 30, and 150, the answer would not have been complete, as the sine of 390 and 410 is also 1/2. These values are greater then 360, but since 2x = 390 or 410, we divide them by 2 to get values that are less then 360.

We could add 360 once more: 30 + 360 + 360 = 750. sin 30 = 1/2 so sine 750 = 1/2. sin (2x) = 1/2 so sin (750) = sin (2x)   so 2x = 750  x = 375.  375 is the exact same angle as 15 degrees. It just spun around in a full circle. Basicly we stop when the values of x get higher then 360.

Hope that helped.

---

A logarithm is just a misspelled algorithm.

mikau

Member

Registered: 2005-08-22

Posts: 1,504

Re: Circular Functions!!!

Here, maybe this can help you.  Remember the 30 60 90 degree triangle. These can be used to find the sines, cosines and tangents of 30, 60, 120, 150, 210, 240, 300, and 330. Look at the diagram. Just remember that the large angle that isn't the 90 degree angle, is the 60 degree angle, and the short one is the 30 degree angle. Using this diagram, you can easily find where the angles stand at. For instance, in the left diagram, the angle  at the top left (the one pointing up and to the right) is 60 degree's before the 180 degree line, therefore its angle is 180 - 60 or 120. The sine = opposite over hypotenuse, or vertical height over hypotenuse. So the sine of 120 = sqrt (3) / 2.

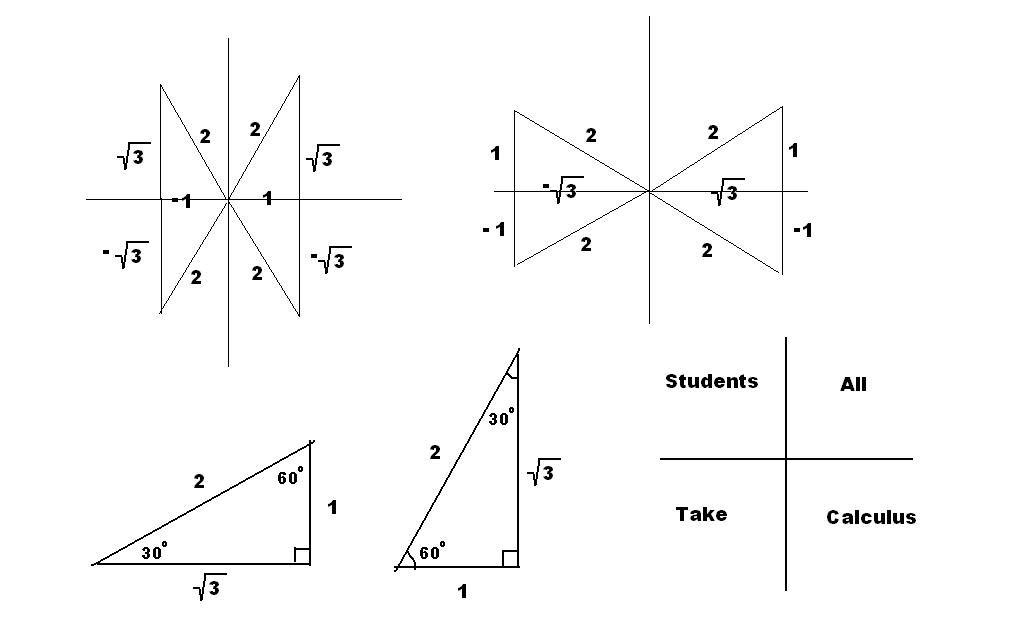
Notice the vertical height is negative when it falls below the origin, and the horizontal lengths are negative when they are to the left of the origin. But the hypotenuse is always postive. Therefore, the cosine of 120 equals hypotenuse over adjacent, or hypotenuse over horizontal length. Here the horizontal length is -1, the hypotenuse is 2 (always positive) so the cosine is -1 over 2 or -1/2. Notice the angle below also has a cosine of -1 over 2.

In the first quadrant, sines, cosines and tangents are all positive. In the second, only sines are postive, in the third quadrant, only tangents are postive, and in the forth quadrant, only cosines are positive. You can use the mnemonic All Students Take Calculus to remember which trigonometric functions are positive in each quadrant. All in the first quadrant all trig functions are positive. Students, S for Sine, in the 2nd quadrant only sines arep positeve. Take, T for tangent, in the third quadrant only tangents are postive. Calculus. C for Cosine, in the 4th quadrant, only cosines are positive.

Hope this helps.

Last edited by mikau (2006-03-05 11:45:05)

---

A logarithm is just a misspelled algorithm.