Math Is Fun Forum

renjer

Member

Registered: 2006-04-29

Posts: 50

Line Integral of a Helix from Point A to B

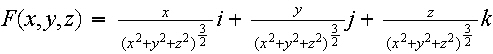
is a vector field. Now how do I evaluate the line integral F.dr in the region C where C starts at the point A(12,0,0), ends at point B (0,12,5) and is an arc on the helix:

x=12cos πt
y=12sin πt
z=2t

where π is the number pi.

I know how to evaluate line integrals for a helix and using F but now with the points added in (point A and B) I have no idea how to begin. Please help.

Ricky

Moderator

Registered: 2005-12-04

Posts: 3,791

Re: Line Integral of a Helix from Point A to B

First, find the limits of integration.   That is, find the start and endpoints of your path.

A = (12,0,0) B = (0,12,5)

x=12cos(pi*t)
y=12sin(pi*t)
z=2t

So we want (12cos(pi*t), 12sin(pi*t), 2t) = (12, 0, 0).  So it must be that 2t = 0.  Thus, t = 0.  Try the other ones, and you'll see that they work as well.

Now we want (12cos(pi*t), 12sin(pi*t), 2t) = (0, 12, 5).  Again, 2t = 5, so t = 5/2.  You can check the others, but it will work out.

So we know that 0 <= t <= 5/2.  These are the limits of integration.

Remember that:

So we need to find F(c(t)) and c'(t).  Once you find these, take the dot product, and then integrate.

---

"In the real world, this would be a problem.  But in mathematics, we can just define a place where this problem doesn't exist.  So we'll go ahead and do that now..."