Math Is Fun Forum
You are not logged in.
- Topics: Active | Unanswered
Pages: 1
#1 2022-10-10 03:39:59
- Jai Ganesh
- Administrator

- Registered: 2005-06-28
- Posts: 51,422
Numbers - Hierarchy
Names of large numbers - Prefixes
This article lists and discusses the usage and derivation of names of large numbers, together with their possible extensions.
Two naming scales have been used in English and other European languages since the early modern era – the long and short scales. Most English variants use the short scale today, but the long scale remains dominant in many non-English-speaking areas, including continental Europe and Spanish-speaking countries in Latin America. These naming procedures are based on taking the number n occurring in
(short scale) or (long scale) and concatenating Latin roots for its units, tens, and hundreds place, together with the suffix -illion.Names of numbers above a trillion are rarely used in practice; such large numbers have practical usage primarily in the scientific domain, where powers of ten are expressed as 10 with a numeric superscript.
Indian English does not use millions, but has its own system of large numbers including lakhs and crores. English also has many words, such as "zillion", used informally to mean large but unspecified amounts.
Standard dictionary numbers
It appears to me that if one wants to make progress in mathematics, one should study the masters and not the pupils. - Niels Henrik Abel.
Nothing is better than reading and gaining more and more knowledge - Stephen William Hawking.
Offline
#2 2022-10-10 13:24:26
- Jai Ganesh
- Administrator

- Registered: 2005-06-28
- Posts: 51,422
Re: Numbers - Hierarchy
Large Numbers
Large numbers are numbers above one million that are usually represented either with the use of an exponent such as
or by terms such as billion or thousand millions that frequently differ from system to system. The American system of numeration for denominations above one million was modeled on a French system, but in 1948 the French system was changed to correspond to the German and British systems. In the American system each of the denominations above 1,000 millions (the American billion) is 1,000 times the preceding one (one trillion = 1,000 billions; one quadrillion = 1,000 trillions). In the British system each of the denominations is 1,000,000 times the preceding one (one trillion = 1,000,000 billions) with the sole exception of milliard, which is sometimes used for 1,000 millions. In recent years British usage has reflected widespread and increasing use of the American system.Have you ever wondered what number comes after a trillion? Or how many zeros there are in a vigintillion? Some day you might need to know this for a science or math class, or if you happen to enter one of several mathematical or scientific fields.
Numbers Bigger Than a Trillion
The digit zero plays an important role as you count very large numbers. It helps to track these multiples of 10 because the larger the number is, the more zeros are needed.
It appears to me that if one wants to make progress in mathematics, one should study the masters and not the pupils. - Niels Henrik Abel.
Nothing is better than reading and gaining more and more knowledge - Stephen William Hawking.
Offline
#3 2022-10-10 13:39:10
- Jai Ganesh
- Administrator

- Registered: 2005-06-28
- Posts: 51,422
Re: Numbers - Hierarchy
Have a look at these Ginormous Numbers:

Googol
A googol is the large number
. In decimal notation, it is written as the digit 1 followed by one hundred zeroes: 10,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000.Etymology
The term was coined in 1920 by 9-year-old Milton Sirotta (1911–1981), nephew of U.S. mathematician Edward Kasner. He may have been inspired by the contemporary comic strip character Barney Google. Kasner popularized the concept in his 1940 book Mathematics and the Imagination. Other names for this quantity include ten duotrigintillion on the short scale, ten thousand sexdecillion on the long scale, or ten sexdecilliard on the Peletier long scale.
Size
A googol has no special significance in mathematics. However, it is useful when comparing with other very large quantities such as the number of subatomic particles in the visible universe or the number of hypothetical possibilities in a chess game. Kasner used it to illustrate the difference between an unimaginably large number and infinity, and in this role it is sometimes used in teaching mathematics.
It appears to me that if one wants to make progress in mathematics, one should study the masters and not the pupils. - Niels Henrik Abel.
Nothing is better than reading and gaining more and more knowledge - Stephen William Hawking.
Offline
#4 2022-10-14 22:06:47
- Jai Ganesh
- Administrator

- Registered: 2005-06-28
- Posts: 51,422
Re: Numbers - Hierarchy
Centillion
Celtillion a cardinal number represented in the U.S. by 1 followed by 303 zeros, and in Great Britain by 1 followed by 600 zeros.
It appears to me that if one wants to make progress in mathematics, one should study the masters and not the pupils. - Niels Henrik Abel.
Nothing is better than reading and gaining more and more knowledge - Stephen William Hawking.
Offline
#5 2022-10-15 02:58:39
- Jai Ganesh
- Administrator

- Registered: 2005-06-28
- Posts: 51,422
Re: Numbers - Hierarchy
Skewes's number
Part - I
In number theory, Skewes's number is any of several large numbers used by the South African mathematician Stanley Skewes as upper bounds for the smallest natural number
for which,where is the prime-counting function and li is the logarithmic integral function. Skewes's number is much larger, but it is now known that there is a crossing near It is not known whether it is the smallest.
It appears to me that if one wants to make progress in mathematics, one should study the masters and not the pupils. - Niels Henrik Abel.
Nothing is better than reading and gaining more and more knowledge - Stephen William Hawking.
Offline
#6 2022-10-24 22:15:47
- Jai Ganesh
- Administrator

- Registered: 2005-06-28
- Posts: 51,422
Re: Numbers - Hierarchy
Skewes's number
Part - II
Skewes's numbers
John Edensor Littlewood, who was Skewes's research supervisor, had proved in Littlewood (1914) that there is such a number (and so, a first such number); and indeed found that the sign of the difference
Skewes (1933) proved that, assuming that the Riemann hypothesis is true, there exists a number x violating
belowIn Skewes (1955), without assuming the Riemann hypothesis, Skewes proved that there must exist a value of x below
Skewes's task was to make Littlewood's existence proof effective: exhibiting some concrete upper bound for the first sign change. According to Georg Kreisel, this was at the time not considered obvious even in principle.
It appears to me that if one wants to make progress in mathematics, one should study the masters and not the pupils. - Niels Henrik Abel.
Nothing is better than reading and gaining more and more knowledge - Stephen William Hawking.
Offline
#7 2023-01-02 13:33:31
- Jai Ganesh
- Administrator

- Registered: 2005-06-28
- Posts: 51,422
Re: Numbers - Hierarchy
Googolplex
A googolplex is the number
, or equivalently, or . Written out in ordinary decimal notation, it is 1 followed by zeroes; that is, a 1 followed by a googol of zeroes.History
In 1920, Edward Kasner's nine-year-old nephew, Milton Sirotta, coined the term googol, which is
, and then proposed the further term googolplex to be "one, followed by writing zeroes until you get tired". Kasner decided to adopt a more formal definition because "different people get tired at different times and it would never do to have Carnera [be] a better mathematician than Dr. Einstein, simply because he had more endurance and could write for longer". It thus became standardized to , due to the right-associativity of exponentiation.Size
A typical book can be printed with
zeros (around 400 pages with 50 lines per page and 50 zeros per line). Therefore, it requires such books to print all the zeros of a googolplex (that is, printing a googol zeros). If each book had a mass of 100 grams, all of them would have a total mass of kilograms. In comparison, Earth's mass is kilograms, the mass of the Milky Way galaxy is estimated at kilograms, and the total mass of all the stars in the observable universe is estimated at .To put this in perspective, the mass of all such books required to write out a googolplex would be vastly greater than the masses of the Milky Way and the Andromeda galaxies combined (by a factor of roughly
), and greater than the mass of the observable universe by a factor of roughly .In pure mathematics
In pure mathematics, there are several notational methods for representing large numbers by which the magnitude of a googolplex could be represented, such as tetration, hyperoperation, Knuth's up-arrow notation, Steinhaus–Moser notation, or Conway chained arrow notation.
It appears to me that if one wants to make progress in mathematics, one should study the masters and not the pupils. - Niels Henrik Abel.
Nothing is better than reading and gaining more and more knowledge - Stephen William Hawking.
Offline
#8 2023-11-23 14:23:13
- Jai Ganesh
- Administrator

- Registered: 2005-06-28
- Posts: 51,422
Re: Numbers - Hierarchy
Tetration
Introduction
The first four hyperoperations are shown here, with tetration being considered the fourth in the series. The unary operation succession, defined as
a'=a+1, is considered to be the zeroth operation.
Addition
n copies of 1 added to a combined by succession.
Multiplication
n copies of a combined by addition.
Exponentiation
n copies of a combined by multiplication.
Tetration
n copies of a combined by exponentiation, right-to-left.
Note that nested exponents are conventionally interpreted from the top down:
means and notIt appears to me that if one wants to make progress in mathematics, one should study the masters and not the pupils. - Niels Henrik Abel.
Nothing is better than reading and gaining more and more knowledge - Stephen William Hawking.
Offline
#9 2024-04-01 09:14:57
- Europe2048
- Member
- Registered: 2024-01-03
- Posts: 41
Re: Numbers - Hierarchy
The study of big numbers is also called "googology".
Offline
#10 2024-04-01 18:32:21
- Jai Ganesh
- Administrator

- Registered: 2005-06-28
- Posts: 51,422
Re: Numbers - Hierarchy
I checked up.
Googology: Googology is the study of nomenclature and properties of large numbers.
It appears to me that if one wants to make progress in mathematics, one should study the masters and not the pupils. - Niels Henrik Abel.
Nothing is better than reading and gaining more and more knowledge - Stephen William Hawking.
Offline
#11 2024-12-24 22:16:53
- Echo_23
- Novice
- Registered: 2024-12-23
- Posts: 6
Re: Numbers - Hierarchy
Names of large numbers - Prefixes
This article lists and discusses the usage and derivation of names of large numbers, together with their possible extensions.
Two naming scales have been used in English and other European languages since the early modern era – the long and short scales. Most English variants use the short scale today, but the long scale remains dominant in many non-English-speaking areas, including continental Europe and Spanish-speaking countries in Latin America. These naming procedures are based on taking the number n occurring in
(short scale) or (long scale) and concatenating Latin roots for its units, tens, and hundreds place, together with the suffix -illion.Names of numbers above a trillion are rarely used in practice; such large numbers have practical usage primarily in the scientific domain, where powers of ten are expressed as 10 with a numeric superscript.
Indian English does not use millions, but has its own system of large numbers including lakhs and crores. English also has many words, such as "zillion", used informally to mean large but unspecified amounts.
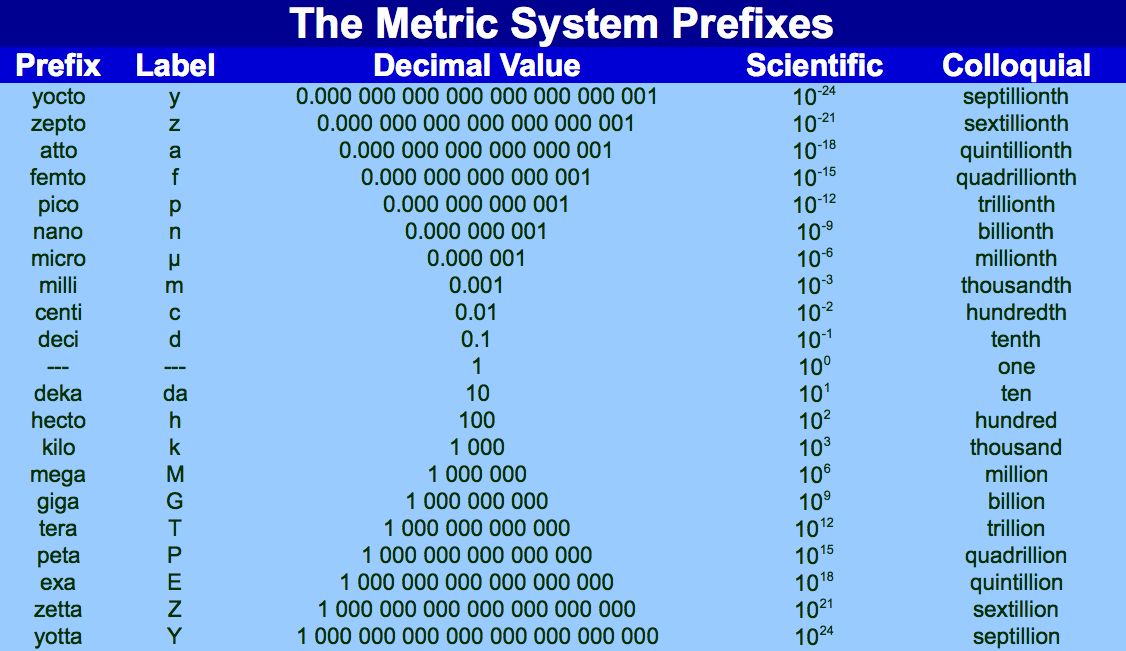
Standard dictionary numbers
http://astro.unl.edu/classaction/tables/intro/si_prefixes.jpg
It is interesting how these systems have evolved and how their use varies across languages and regions. The short scale, which is common in English-speaking countries, simplifies the situation somewhat, but the long scale (used in some European and Latin American countries) can cause some confusion when comparing data internationally.
Offline
#12 2024-12-24 22:46:48
- Jai Ganesh
- Administrator

- Registered: 2005-06-28
- Posts: 51,422
Re: Numbers - Hierarchy
Long and short scales
The long and short scales are two of several naming systems for integer powers of ten which use some of the same terms for different magnitudes.
Some languages, particularly in East Asia and South Asia, have large number naming systems that are different from both the long and short scales, such as the Indian numbering system and the Chinese, Japanese, or Korean numerals.
Much of the remainder of the world adopted either the short scale or the long scale for everyday counting powers of ten. Countries with the usage of the long scale include most countries in continental Europe and most that are French-speaking, German-speaking and Spanish-speaking. Usage of the short scale is found in Brazil and in most English-speaking and Arabic-speaking countries.
For whole numbers smaller than 1,000,000,000 ({10}^{9}), such as one thousand or one million, the two scales are identical. For larger numbers, starting with {10}^{9}, the two systems differ. For identical names, the long scale proceeds by powers of one million, whereas the short scale proceeds by powers of one thousand. For example, the short scale "one billion" (in many languages other than English called "one milliard", even on the short scale) means one thousand million (1,000,000,000), whereas in the long scale, "one billion" always means one million million (1,000,000,000,000). The long scale system employs additional terms for interleaved values, typically replacing the word ending "-ion" by "-iard". For numbers from {10}^{12} and up, all the names in the short scale have different meanings than they have in the long scale.
To avoid confusion resulting from the coexistence of the two scales, the International System of Units (SI) recommends using the metric prefix to indicate orders of magnitude, associated with physical quantities.
Scales
In both short and long scale naming, names are given to each multiplication step for increments of the base-10 exponent of three, i.e. for each integer n in the sequence of multipliers {10}^{3n}. Both systems use the same names for certain multipliers, including those for all numbers smaller than {10}^{9}. The differences arise from the assignment of identical names to specific values of n, for numbers starting with {10}^{9}, for which n=3. In the short scale system, the identical names are for n=3, 4, 5, ..., while the long scale places them at n=4, 6, 8, etc.
Short scale
In the short scale, a billion (in many countries called a "milliard") means a thousand millions (1,000,000,000, which is {10}^{9}), a trillion means one thousand billions ({10}^{12}), and so on. Thus, a short scale n-illion equals {10}^{3n+3}.
Long scale
In the long scale, a billion means one million millions ({10}^{12}) and a trillion means one million billions ({10}^{18}), and so on. Therefore, a long scale n-illion equals {10}^{6n}.
In some languages, the long scale uses additional names for the intermediate multipliers, replacing the ending -ion with -iard; for example, the next multiplier after million is milliard ({10}^{9}); after a billion it is billiard ({10}^{15}). Hence, a long scale n-iard equals {10}^{6n+3}.
It appears to me that if one wants to make progress in mathematics, one should study the masters and not the pupils. - Niels Henrik Abel.
Nothing is better than reading and gaining more and more knowledge - Stephen William Hawking.
Offline
#13 2024-12-25 05:57:52
- KerimF
- Member
- From: Aleppo-Syria
- Registered: 2018-08-10
- Posts: 294
Re: Numbers - Hierarchy
Therefore, the population on earth is expressed by milliard in both scales.
Every living thing has no choice but to execute its pre-programmed instructions embedded in it (known as instincts).
But only a human may have the freedom and ability to oppose his natural robotic nature.
But, by opposing it, such a human becomes no more of this world.
Offline
#14 2024-12-25 07:46:45
- Jai Ganesh
- Administrator

- Registered: 2005-06-28
- Posts: 51,422
Re: Numbers - Hierarchy
Yes; Earth's population can be a little more than 8 milliard or more, at present.
It appears to me that if one wants to make progress in mathematics, one should study the masters and not the pupils. - Niels Henrik Abel.
Nothing is better than reading and gaining more and more knowledge - Stephen William Hawking.
Offline
Pages: 1