Math Is Fun Forum

George,Y

Member

Registered: 2006-03-12

Posts: 1,379

Sum of an Infinite Series

---

X'(y-Xβ)=0

Zmurf

Member

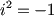
Registered: 2005-07-31

Posts: 49

Re: Sum of an Infinite Series

Couldn't that be re-written as:

I havn't had much experience with lim, and can't remember what it signifys. What exactly is your question?

Edit: Upon refreshing my memories with limits (The almighty Wikipedia ) The formula I specified is incorrect. I don't know if you already know what your formula does or not. Could supply a bit more information? As far as I can tell, your forumla is getting as close to zero as possible and then bigger at a slower rate depending on the size of n.

If you were to make n = 900. It would make the sum of:

Where i was increasing from 1 until it reached 900.

Last edited by Zmurf (2006-05-14 19:18:31)

---

"When subtracted from 180, the sum of the square-root of the two equal angles of an isocoles triangle squared will give the square-root of the remaining angle squared."

krassi_holmz

Real Member

Registered: 2005-12-02

Posts: 1,905

Re: Sum of an Infinite Series

Good.
The interesting here is that n is in non-trivial place in the sum.

---

IPBLE:  Increasing Performance By Lowering Expectations.

krassi_holmz

Real Member

Registered: 2005-12-02

Posts: 1,905

Re: Sum of an Infinite Series

...I think...:)

---

IPBLE:  Increasing Performance By Lowering Expectations.

MathsIsFun

Administrator

Registered: 2005-01-21

Posts: 7,713

Re: Sum of an Infinite Series

From Excel. Notice the interesting pattern. This may help us rewrite the equation.

---

"The physicists defer only to mathematicians, and the mathematicians defer only to God ..."  - Leon M. Lederman

krassi_holmz

Real Member

Registered: 2005-12-02

Posts: 1,905

Re: Sum of an Infinite Series

A plot:

Last edited by krassi_holmz (2006-05-14 20:28:39)

---

IPBLE:  Increasing Performance By Lowering Expectations.

George,Y

Member

Registered: 2006-03-12

Posts: 1,379

Re: Sum of an Infinite Series

Good.
The interesting here is that n is in non-trivial place in the sum.

Yes, you are smart!

To Zmurf and Krassi:
Originally it's a Rieman Sum, an integration question. So I guess Krassi has used integral.

Original Question:

Last edited by George,Y (2006-05-15 15:21:13)

---

X'(y-Xβ)=0

krassi_holmz

Real Member

Registered: 2005-12-02

Posts: 1,905

Re: Sum of an Infinite Series

???
That's A Limit!!!

---

IPBLE:  Increasing Performance By Lowering Expectations.

George,Y

Member

Registered: 2006-03-12

Posts: 1,379

Re: Sum of an Infinite Series

Yes, and that does return a limit result log2

---

X'(y-Xβ)=0

liuv

Member

Registered: 2006-05-14

Posts: 29

Re: Sum of an Infinite Series

---

I'm from Beijing China.

George,Y

Member

Registered: 2006-03-12

Posts: 1,379

Re: Sum of an Infinite Series

How do you transform it into a Rieman Sum? There is no Δx or another 1/n in it!

The integral is indeed a limit if you admit the property of Delta Function:

where

Last edited by George,Y (2006-05-20 21:16:17)

---

X'(y-Xβ)=0

krassi_holmz

Real Member

Registered: 2005-12-02

Posts: 1,905

Re: Sum of an Infinite Series

Here's a SUM (I actually need it for a question):
Find:

,
where P means the prime number set.

---

IPBLE:  Increasing Performance By Lowering Expectations.

MathsIsFun

Administrator

Registered: 2005-01-21

Posts: 7,713

Re: Sum of an Infinite Series

The sum of the reciprocal of every prime to power n?

Download some primes from here: Prime Number List

Then put into Excel.

For Primes up to 100,000
n=1: 2.705272179
n=2: 0.452246618
n=10: 0.000993604
n=20: 9.53961E-07

So that seems to be heading for 0, but then there are infinitely many primes, not just the 9,500 in the list.

(The "2.70" for n=1 is interesting)

(Here's another thought, it will be less than the same sum over positive integers)

---

"The physicists defer only to mathematicians, and the mathematicians defer only to God ..."  - Leon M. Lederman

liuv

Member

Registered: 2006-05-14

Posts: 29

Re: Sum of an Infinite Series

oh...my English is poor..so i can not express what i think sometimes.:D but i'm very sure my answer is right.:P

on the image:

Last edited by liuv (2006-05-21 02:46:48)

---

I'm from Beijing China.

George,Y

Member

Registered: 2006-03-12

Posts: 1,379

Re: Sum of an Infinite Series

Thank you liuv, good shifting! My thought is

for krassi holmz,
you may check out some properties for prime numbers.
PS> how did you put "n->∞" under "lim"?

Last edited by George,Y (2006-05-22 02:31:16)

---

X'(y-Xβ)=0

Ricky

Moderator

Registered: 2005-12-04

Posts: 3,791

Re: Sum of an Infinite Series

Like that.  Basically, put a \ before lim.

---

"In the real world, this would be a problem.  But in mathematics, we can just define a place where this problem doesn't exist.  So we'll go ahead and do that now..."

krassi_holmz

Real Member

Registered: 2005-12-02

Posts: 1,905

Re: Sum of an Infinite Series

Yep.

\lim_{n \to \infty}

---

IPBLE:  Increasing Performance By Lowering Expectations.

krassi_holmz

Real Member

Registered: 2005-12-02

Posts: 1,905

Re: Sum of an Infinite Series

but then there are infinitely many primes, not just the 9,500 in the list.

That's my point that you can't compute the lim directly- for different number of the primes you'll get different result.

---

IPBLE:  Increasing Performance By Lowering Expectations.

George,Y

Member

Registered: 2006-03-12

Posts: 1,379

Re: Sum of an Infinite Series

thanks a lot!

---

X'(y-Xβ)=0